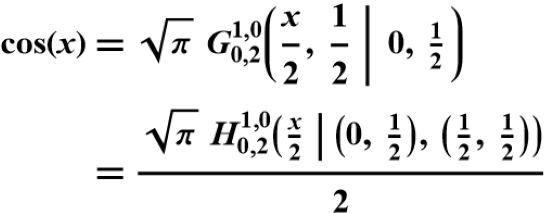Hello, r/badmathematics! Today I will present you a (surprisingly rare) badmathematics from the beloved Facebook group Mathematical Mathematics Memes. The badmaths in question is not the Facebook post itself, but rather the bizarre replies to one of the comments in this post.

We start with a comment asking for the proof of the recent aperiodic monotiling, with a joking mention of accepting it like Euclid's fifth postulate.

The replies are, however, something else:

Of course, anyone who had taken a course on set theory knows immediately that this is false. The statement "1+1=2" is probably proved every time it is taught, starting with the discussion of Peano axioms and whatnot. Furthermore, Principia Mathematica did in fact prove it, not just merely mention it in passing. The badmaths-er's point that 2 is simply defined as 1+1 is also inaccurate. 2 is defined as the successor of 1, and it takes a bit of work (albeit just unpacking the definition) that 1+1 = 2.^{Foreshadowing...}

I would go a bit further and digress that, most mathematicians who are not diehard logicism fans don't prove "1+ 1= 2" to believe that indeed 1+ 1= 2. The proof is just a mathematical sanity check that their mathematical systems of ZFC/Peano are working as intended and agree with their knowledge that 1+1=2.

There is also some nonsense about units. The moment when you take two animals and count the total number of legs, you are not doing a mathematical addition anymore, and so is not a contradiction to mathematics.

And for the last paragraph, no, that's not what the incompleteness theorems are about. A proof by contradiction is a valid rule of inference in classical logic and hence works in systems with or without consistency. (If it is inconsistent then we can prove anything anyways.) We care about the soundness of systems when we want our theorems to be true, and the incompleteness theorems say nothing about soundness, but rather that we cannot tell that within the systems themself. The badmaths is conflating the provability and truth of a statement.

In the next replies, the badmaths-er is implying that the successor of numbers is a mistaken notion, and taking a limit is evaluating [the expressions?] at the true successor, whatever that means. Obviously, this is not how we think about limits and the successor function. It seems like he is confused about infinitesimal, which is often told as "the smallest number next to zero" and the successor function of a natural number.

Extra sweet is the implication that modern mathematics is taught by people with agenda, from Big Logic probably.

Classic conflation about two different meanings of the word "number". It is true that 2 is the natural number after 1, but 1.5 and phi are real numbers, not natural numbers. No contradiction here.

Wow, the first statement is already false, and the "for instance" does not relate to it in any way. Units might be useful when applying mathematics to sciences, but it is not how mathematicians think of numbers as a unitless, abstract quantity, or an element of some particular sets.

It seems like the poster tries to define the "true" successor as the infinitesimal number next to a number. You change an established terminology and old usage no longer matches, mathematics must be wrong! Also, the largest possible infinity does not make sense as a limit on the extended real line, such a notion does not exist. And again Gödel said nothing about successor function being a wrong approach.

Of course, it goes without saying that 1 is not aleph-nought. His claim is not-even-wrong, simply put. And anyone who dares to disagree is just blindly following dogma.

But why is 1/0 simultaneously the largest possible infinity, and is still aleph-null, which is smaller than 1=aleph-naught? Nobody can answer this conundrum.

In the last reply before the banhammer strike, the badmaths-er claims that 6 and -6 are not numbers, because they "have the same number component". It seems like he regards the minus sign as an indication of directions in a physical, vectorial sense. Numbers are not vectors, and signs did not convey a sense of direction. In elementary physics, you first fix a reference frame or direction, only then the sign of a number has a meaning as an indication of direction. Note again this is how mathematics is applied, with physics' conventions etc., and not how mathematicians conceive of numbers.

This last one is from his participation answers taken from the moderators. Mathematics is in shambles.