- published: 12 May 2009
- views: 31024
-
remove the playlistCurl (mathematics)
-
remove the playlistLongest Videos
- remove the playlistCurl (mathematics)
- remove the playlistLongest Videos
- published: 12 Aug 2008
- views: 158401
- published: 12 Aug 2008
- views: 77750
- published: 26 Jul 2011
- views: 42365
- published: 26 Jul 2011
- views: 47170
- published: 26 Jul 2011
- views: 70205
- published: 14 Apr 2013
- views: 4915
- published: 10 Oct 2015
- views: 1749
- published: 08 Nov 2015
- views: 2883
- published: 11 Oct 2015
- views: 1798
- published: 22 Jan 2016
- views: 2

In vector calculus, the curl is a vector operator that describes the infinitesimal rotation of a 3-dimensional vector field. At every point in the field, the curl of that field is represented by a vector. The attributes of this vector (length and direction) characterize the rotation at that point.
The direction of the curl is the axis of rotation, as determined by the right-hand rule, and the magnitude of the curl is the magnitude of rotation. If the vector field represents the flow velocity of a moving fluid, then the curl is the circulation density of the fluid. A vector field whose curl is zero is called irrotational. The curl is a form of differentiation for vector fields. The corresponding form of the fundamental theorem of calculus is Stokes' theorem, which relates the surface integral of the curl of a vector field to the line integral of the vector field around the boundary curve.
The alternative terminology rotor or rotational and alternative notations rot F and ∇×F are often used (the former especially in many European countries, the latter, using the del operator and the cross product, is more used in other countries) for curl and curl F.
This article is licensed under the Creative Commons Attribution-ShareAlike 3.0 Unported License, which means that you can copy and modify it as long as the entire work (including additions) remains under this license.
Curl or CURL may refer to:
In technology
Other
This article is licensed under the Creative Commons Attribution-ShareAlike 3.0 Unported License, which means that you can copy and modify it as long as the entire work (including additions) remains under this license.

Mathematics (from Greek μάθημα máthēma, “knowledge, study, learning”) is the study of quantity, structure, space, and change.Mathematicians seek out patterns and formulate new conjectures. Mathematicians resolve the truth or falsity of conjectures by mathematical proof. The research required to solve mathematical problems can take years or even centuries of sustained inquiry. Since the pioneering work of Giuseppe Peano (1858–1932), David Hilbert (1862–1943), and others on axiomatic systems in the late 19th century, it has become customary to view mathematical research as establishing truth by rigorous deduction from appropriately chosen axioms and definitions. When those mathematical structures are good models of real phenomena, then mathematical reasoning often provides insight or predictions.
Through the use of abstraction and logical reasoning, mathematics developed from counting, calculation, measurement, and the systematic study of the shapes and motions of physical objects. Practical mathematics has been a human activity for as far back as written records exist. Rigorous arguments first appeared in Greek mathematics, most notably in Euclid's Elements. Mathematics developed at a relatively slow pace until the Renaissance, when mathematical innovations interacting with new scientific discoveries led to a rapid increase in the rate of mathematical discovery that has continued to the present day.
This article is licensed under the Creative Commons Attribution-ShareAlike 3.0 Unported License, which means that you can copy and modify it as long as the entire work (including additions) remains under this license.
- Loading...

-
 48:35
48:35What is the Curl? Chris Tisdell UNSW
What is the Curl? Chris Tisdell UNSWWhat is the Curl? Chris Tisdell UNSW
This lecture gently introduces the idea of a the "curl" of a vector field. The curl is one of the basic operations of vector calculus. Dr Chris Tisdell discusses the definition of the curl and how to compute it. Plently of examples are provided. A physical interpretation of the curl is also presented in terms of circulation density. Basically speaking, curl measures the tendency of a vector field to "swirl" around a point. -
 9:33
9:33Curl 1 | Partial derivatives, gradient, divergence, curl | Multivariable Calculus | Khan Academy
Curl 1 | Partial derivatives, gradient, divergence, curl | Multivariable Calculus | Khan AcademyCurl 1 | Partial derivatives, gradient, divergence, curl | Multivariable Calculus | Khan Academy
Introduction to the curl of a vector field Watch the next lesson: https://www.khanacademy.org/math/multivariable-calculus/partial_derivatives_topic/curl/v/curl-2?utm_source=YT&utm;_medium=Desc&utm;_campaign=MultivariableCalculus Missed the previous lesson? https://www.khanacademy.org/math/multivariable-calculus/partial_derivatives_topic/divergence/v/divergence-3?utm_source=YT&utm;_medium=Desc&utm;_campaign=MultivariableCalculus Multivariable Calculus on Khan Academy: Think calculus. Then think algebra II and working with two variables in a single equation. Now generalize and combine these two mathematical concepts, and you begin to see some of what Multivariable calculus entails, only now include multi dimensional thinking. Typical concepts or operations may include: limits and continuity, partial differentiation, multiple integration, scalar functions, and fundamental theorem of calculus in multiple dimensions. About Khan Academy: Khan Academy offers practice exercises, instructional videos, and a personalized learning dashboard that empower learners to study at their own pace in and outside of the classroom. We tackle math, science, computer programming, history, art history, economics, and more. Our math missions guide learners from kindergarten to calculus using state-of-the-art, adaptive technology that identifies strengths and learning gaps. We've also partnered with institutions like NASA, The Museum of Modern Art, The California Academy of Sciences, and MIT to offer specialized content. For free. For everyone. Forever. #YouCanLearnAnything Subscribe to KhanAcademy’s Multivariable Calculus channel: https://www.youtube.com/channel/UCQQZDc22yCogOyx6DwXt-Ig?sub_confirmation=1 Subscribe to KhanAcademy: https://www.youtube.com/subscription_center?add_user=khanacademy -
 10:23
10:23Curl 3 | Partial derivatives, gradient, divergence, curl | Multivariable Calculus | Khan Academy
Curl 3 | Partial derivatives, gradient, divergence, curl | Multivariable Calculus | Khan AcademyCurl 3 | Partial derivatives, gradient, divergence, curl | Multivariable Calculus | Khan Academy
More on curl Watch the next lesson: https://www.khanacademy.org/math/multivariable-calculus/line_integrals_topic/line_integrals/v/introduction-to-the-line-integral?utm_source=YT&utm;_medium=Desc&utm;_campaign=MultivariableCalculus Missed the previous lesson? https://www.khanacademy.org/math/multivariable-calculus/partial_derivatives_topic/curl/v/curl-2?utm_source=YT&utm;_medium=Desc&utm;_campaign=MultivariableCalculus Multivariable Calculus on Khan Academy: Think calculus. Then think algebra II and working with two variables in a single equation. Now generalize and combine these two mathematical concepts, and you begin to see some of what Multivariable calculus entails, only now include multi dimensional thinking. Typical concepts or operations may include: limits and continuity, partial differentiation, multiple integration, scalar functions, and fundamental theorem of calculus in multiple dimensions. About Khan Academy: Khan Academy offers practice exercises, instructional videos, and a personalized learning dashboard that empower learners to study at their own pace in and outside of the classroom. We tackle math, science, computer programming, history, art history, economics, and more. Our math missions guide learners from kindergarten to calculus using state-of-the-art, adaptive technology that identifies strengths and learning gaps. We've also partnered with institutions like NASA, The Museum of Modern Art, The California Academy of Sciences, and MIT to offer specialized content. For free. For everyone. Forever. #YouCanLearnAnything Subscribe to KhanAcademy’s Multivariable Calculus channel: https://www.youtube.com/channel/UCQQZDc22yCogOyx6DwXt-Ig?sub_confirmation=1 Subscribe to KhanAcademy: https://www.youtube.com/subscription_center?add_user=khanacademy -
 7:07
7:07Grad - Grad, Div and Curl (1/3)
Grad - Grad, Div and Curl (1/3)Grad - Grad, Div and Curl (1/3)
For more like this subscribe to the Open University channel https://www.youtube.com/channel/UCXsH4hSV_kEdAOsupMMm4Qw Free learning from The Open University http://www.open.ac.uk/openlearn/science-maths-technology/mathematics-and-statistics --- Introduction to this gradient vector. What is meant by 'steepness of a path' on a hillside. (Part 1 of 3) Playlist link - http://www.youtube.com/playlist?list=PLB4CDD2976B5FE747 Transcript link - http://media-podcast.open.ac.uk/feeds/mst209-grad-div-curl/transcript/mst207grad01.pdf --- For more information about Grad visit http://www3.open.ac.uk/study/undergraduate/course/mst209.htm -
 7:32
7:32Div - Grad, Div and Curl (2/3)
Div - Grad, Div and Curl (2/3)Div - Grad, Div and Curl (2/3)
For more like this subscribe to the Open University channel https://www.youtube.com/channel/UCXsH4hSV_kEdAOsupMMm4Qw Free learning from The Open University http://www.open.ac.uk/openlearn/science-maths-technology/mathematics-and-statistics --- Introduction to the divergence div. Heat flow and temperature distribution inside a modern nuclear reactor. (Part 2 of 3) Playlist link - http://www.youtube.com/playlist?list=PLB4CDD2976B5FE747 Transcript link - http://media-podcast.open.ac.uk/feeds/mst209-grad-div-curl/transcript/mst207grad02.pdf --- For more information about Div visit http://www3.open.ac.uk/study/undergraduate/course/mst209.htm -
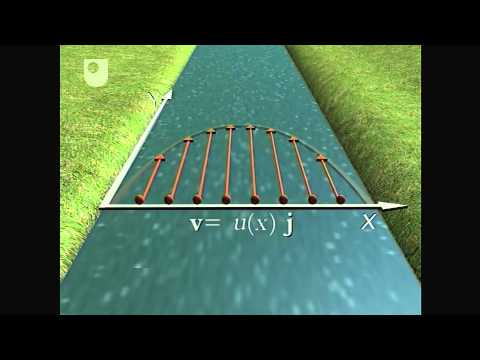 10:28
10:28Curl - Grad, Div and Curl (3/3)
Curl - Grad, Div and Curl (3/3)Curl - Grad, Div and Curl (3/3)
For more like this subscribe to the Open University channel https://www.youtube.com/channel/UCXsH4hSV_kEdAOsupMMm4Qw Free learning from The Open University http://www.open.ac.uk/openlearn/science-maths-technology/mathematics-and-statistics --- Introduction to this vector operation through the context of modelling water flow in a river. How curl helps in predicting storms. (Part 3 of 3) Playlist link - http://www.youtube.com/playlist?list=PLB4CDD2976B5FE747 Transcript link - http://media-podcast.open.ac.uk/feeds/mst209-grad-div-curl/transcript/mst207grad03.pdf --- For more information about Curl visit http://www3.open.ac.uk/study/undergraduate/course/mst209.htm -
 20:15
20:15UPenn Math 114 Introduction to how to Calculate Gradient, Divergence, and Curl
UPenn Math 114 Introduction to how to Calculate Gradient, Divergence, and CurlUPenn Math 114 Introduction to how to Calculate Gradient, Divergence, and Curl
Brief lecture introducing divergence and curl and how they are calculated. -
 13:16
13:16GATE 2016- Engineering Mathematics- CURL of function Calculus part-10
GATE 2016- Engineering Mathematics- CURL of function Calculus part-10GATE 2016- Engineering Mathematics- CURL of function Calculus part-10
This video is for Students who are preparing for GATE (Graduate Aptitude Test in Engineering). This series of videos are detailed free study material for GATE exam. More video will be uploaded later. The aim of the video is to provide solid concept of the topic so that you can solve any questions asked in gate exam in that topic. http://3hindi.com/ -
 56:03
56:03Divergence and Curl of a vector point function in Hindi
Divergence and Curl of a vector point function in HindiDivergence and Curl of a vector point function in Hindi
This video lecture " divergence and curl of vector point function in Hindi" will help Engineering and Basic Science students to understand following topic of of Engineering-Mathematics: 1. Definition and meaning of divergence and curl of vector point function. 2. Solenoidal vector and irrotational vector function. 3. 3 solved problem For any query and feedback, please write us at: jaipal.vishwakarma@gmail.com OR call us at: +919301197409 For latest updates subscribe our channel " Bhagwan Singh Vishwakarma" or join us on Facebook "Maths Bhopal"... -
 5:12
5:12GATE 2016- Engineering Mathematics- grad, curl, div, combined relations part-11
GATE 2016- Engineering Mathematics- grad, curl, div, combined relations part-11GATE 2016- Engineering Mathematics- grad, curl, div, combined relations part-11
This video is for Students who are preparing for GATE (Graduate Aptitude Test in Engineering). This series of videos are detailed free study material for GATE exam. More video will be uploaded later. The aim of the video is to provide solid concept of the topic so that you can solve any questions asked in gate exam in that topic. http://3hindi.com/ -
 28:02
28:02Unit-2 Diverg Curl Problems - Mathematics
Unit-2 Diverg Curl Problems - MathematicsUnit-2 Diverg Curl Problems - Mathematics
http://www.gurug.net Unit-2 Diverg Curl Problems - Mathematics -
 14:52
14:52Curl (mathematics)
Curl (mathematics)Curl (mathematics)
Curl (mathematics) In vector calculus, the curl is a vector operator that describes the infinitesimal rotation of a 3-dimensional vector field.At every point in the field, the curl of that point is represented by a vector. -Video is targeted to blind users Attribution: Article text available under CC-BY-SA image source in video https://www.youtube.com/watch?v=nbDdQhreNDE -
 0:58
0:58ravish On physics of determinants and CURL Meaning of CURL.Mathematical physics
ravish On physics of determinants and CURL Meaning of CURL.Mathematical physicsravish On physics of determinants and CURL Meaning of CURL.Mathematical physics
In this short presentation, the physical meaning of curl is explained .The curl representing the rotation of a vector is shown through diagrams. -
 5:22
5:22Curl of a vector field (ex. no.1): Vector Calculus
Curl of a vector field (ex. no.1): Vector CalculusCurl of a vector field (ex. no.1): Vector Calculus
Free ebook http://tinyurl.com/EngMathYT I present and solve a simple example where the curl of a given vector field is sought. The curl is one of the basic operations of "vector calculus". Such and example is seen in 2nd year university mathematics. Such an example is seen in 2nd year university mathematics courses.
- Area density
- Binomial coefficient
- Bivector
- Calculus
- Chain rule
- Change of variables
- Continuous function
- Cross product
- Curl (mathematics)
- Del
- Derivative
- Differential form
- Disk integration
- Divergence
- Divergence theorem
- Edwin Bidwell Wilson
- Eric W. Weisstein
- Exterior algebra
- Exterior derivative
- Flow velocity
- Fluid
- Gradient
- Gradient theorem
- Green's theorem
- Hodge dual
- Hodge duality
- Improper integral
- Integral
- Integral calculus
- Integration by parts
- Irrotational
- James Clerk Maxwell
- Josiah Willard Gibbs
- Laplace operator
- Levi-Civita symbol
- Limit of a function
- Line integral
- Lists of integrals
- MathWorld
- Matrix calculus
- Maxwell's equations
- Mean value theorem
- Metric tensor
- Mnemonic
- Multiple integral
- Musical isomorphism
- Nondegenerate form
- Operator (physics)
- P-vector
- Paddle wheel
- Partial derivative
- Pascal's triangle
- Physics
- Power rule
- Product rule
- Quotient rule
- Related rates
- Riemannian manifold
- Riemannian metric
- Right-hand rule
- Rotation
- Scalar field
- Shell integration
- Smooth function
- Stokes' theorem
- Surface integral
- Taylor's theorem
- Template Calculus
- Unit vector
- Vector (geometry)
- Vector bundle
- Vector calculus
- Vector cross product
- Vector field
- Vector operator
- Volume integral
- Vorticity
- Zero vector
-

What is the Curl? Chris Tisdell UNSW
This lecture gently introduces the idea of a the "curl" of a vector field. The curl is one of the basic operations of vector calculus. Dr Chris Tisdell discusses the definition of the curl and how to compute it. Plently of examples are provided. A physical interpretation of the curl is also presented in terms of circulation density. Basically speaking, curl measures the tendency of a vector field to "swirl" around a point. -

Curl 1 | Partial derivatives, gradient, divergence, curl | Multivariable Calculus | Khan Academy
Introduction to the curl of a vector field Watch the next lesson: https://www.khanacademy.org/math/multivariable-calculus/partial_derivatives_topic/curl/v/curl-2?utm_source=YT&utm;_medium=Desc&utm;_campaign=MultivariableCalculus Missed the previous lesson? https://www.khanacademy.org/math/multivariable-calculus/partial_derivatives_topic/divergence/v/divergence-3?utm_source=YT&utm;_medium=Desc&utm;_campaign=MultivariableCalculus Multivariable Calculus on Khan Academy: Think calculus. Then think algebra II and working with two variables in a single equation. Now generalize and combine these two mathematical concepts, and you begin to see some of what Multivariable calculus entails, only now include multi dimensional thinking. Typical concepts or operations may include: limits and continuity, partial differentiation, multiple integration, scalar functions, and fundamental theorem of calculus in multiple dimensions. About Khan Academy: Khan Academy offers practice exercises, instructional videos, and a personalized learning dashboard that empower learners to study at their own pace in and outside of the classroom. We tackle math, science, computer programming, history, art history, economics, and more. Our math missions guide learners from kindergarten to calculus using state-of-the-art, adaptive technology that identifies strengths and learning gaps. We've also partnered with institutions like NASA, The Museum of Modern Art, The California Academy of Sciences, and MIT to offer specialized content. For free. For everyone. Forever. #YouCanLearnAnything Subscribe to KhanAcademy’s Multivariable Calculus channel: https://www.youtube.com/channel/UCQQZDc22yCogOyx6DwXt-Ig?sub_confirmation=1 Subscribe to KhanAcademy: https://www.youtube.com/subscription_center?add_user=khanacademy -

Curl 3 | Partial derivatives, gradient, divergence, curl | Multivariable Calculus | Khan Academy
More on curl Watch the next lesson: https://www.khanacademy.org/math/multivariable-calculus/line_integrals_topic/line_integrals/v/introduction-to-the-line-integral?utm_source=YT&utm;_medium=Desc&utm;_campaign=MultivariableCalculus Missed the previous lesson? https://www.khanacademy.org/math/multivariable-calculus/partial_derivatives_topic/curl/v/curl-2?utm_source=YT&utm;_medium=Desc&utm;_campaign=MultivariableCalculus Multivariable Calculus on Khan Academy: Think calculus. Then think algebra II and working with two variables in a single equation. Now generalize and combine these two mathematical concepts, and you begin to see some of what Multivariable calculus entails, only now include multi dimensional thinking. Typical concepts or operations may include: limits and continuity, partial differentiation, multiple integration, scalar functions, and fundamental theorem of calculus in multiple dimensions. About Khan Academy: Khan Academy offers practice exercises, instructional videos, and a personalized learning dashboard that empower learners to study at their own pace in and outside of the classroom. We tackle math, science, computer programming, history, art history, economics, and more. Our math missions guide learners from kindergarten to calculus using state-of-the-art, adaptive technology that identifies strengths and learning gaps. We've also partnered with institutions like NASA, The Museum of Modern Art, The California Academy of Sciences, and MIT to offer specialized content. For free. For everyone. Forever. #YouCanLearnAnything Subscribe to KhanAcademy’s Multivariable Calculus channel: https://www.youtube.com/channel/UCQQZDc22yCogOyx6DwXt-Ig?sub_confirmation=1 Subscribe to KhanAcademy: https://www.youtube.com/subscription_center?add_user=khanacademy -

Grad - Grad, Div and Curl (1/3)
For more like this subscribe to the Open University channel https://www.youtube.com/channel/UCXsH4hSV_kEdAOsupMMm4Qw Free learning from The Open University http://www.open.ac.uk/openlearn/science-maths-technology/mathematics-and-statistics --- Introduction to this gradient vector. What is meant by 'steepness of a path' on a hillside. (Part 1 of 3) Playlist link - http://www.youtube.com/playlist?list=PLB4CDD2976B5FE747 Transcript link - http://media-podcast.open.ac.uk/feeds/mst209-grad-div-curl/transcript/mst207grad01.pdf --- For more information about Grad visit http://www3.open.ac.uk/study/undergraduate/course/mst209.htm -

Div - Grad, Div and Curl (2/3)
For more like this subscribe to the Open University channel https://www.youtube.com/channel/UCXsH4hSV_kEdAOsupMMm4Qw Free learning from The Open University http://www.open.ac.uk/openlearn/science-maths-technology/mathematics-and-statistics --- Introduction to the divergence div. Heat flow and temperature distribution inside a modern nuclear reactor. (Part 2 of 3) Playlist link - http://www.youtube.com/playlist?list=PLB4CDD2976B5FE747 Transcript link - http://media-podcast.open.ac.uk/feeds/mst209-grad-div-curl/transcript/mst207grad02.pdf --- For more information about Div visit http://www3.open.ac.uk/study/undergraduate/course/mst209.htm -

Curl - Grad, Div and Curl (3/3)
For more like this subscribe to the Open University channel https://www.youtube.com/channel/UCXsH4hSV_kEdAOsupMMm4Qw Free learning from The Open University http://www.open.ac.uk/openlearn/science-maths-technology/mathematics-and-statistics --- Introduction to this vector operation through the context of modelling water flow in a river. How curl helps in predicting storms. (Part 3 of 3) Playlist link - http://www.youtube.com/playlist?list=PLB4CDD2976B5FE747 Transcript link - http://media-podcast.open.ac.uk/feeds/mst209-grad-div-curl/transcript/mst207grad03.pdf --- For more information about Curl visit http://www3.open.ac.uk/study/undergraduate/course/mst209.htm -

UPenn Math 114 Introduction to how to Calculate Gradient, Divergence, and Curl
Brief lecture introducing divergence and curl and how they are calculated. -

GATE 2016- Engineering Mathematics- CURL of function Calculus part-10
This video is for Students who are preparing for GATE (Graduate Aptitude Test in Engineering). This series of videos are detailed free study material for GATE exam. More video will be uploaded later. The aim of the video is to provide solid concept of the topic so that you can solve any questions asked in gate exam in that topic. http://3hindi.com/ -

Divergence and Curl of a vector point function in Hindi
This video lecture " divergence and curl of vector point function in Hindi" will help Engineering and Basic Science students to understand following topic of of Engineering-Mathematics: 1. Definition and meaning of divergence and curl of vector point function. 2. Solenoidal vector and irrotational vector function. 3. 3 solved problem For any query and feedback, please write us at: jaipal.vishwakarma@gmail.com OR call us at: +919301197409 For latest updates subscribe our channel " Bhagwan Singh Vishwakarma" or join us on Facebook "Maths Bhopal"... -

GATE 2016- Engineering Mathematics- grad, curl, div, combined relations part-11
This video is for Students who are preparing for GATE (Graduate Aptitude Test in Engineering). This series of videos are detailed free study material for GATE exam. More video will be uploaded later. The aim of the video is to provide solid concept of the topic so that you can solve any questions asked in gate exam in that topic. http://3hindi.com/ -

Unit-2 Diverg Curl Problems - Mathematics
http://www.gurug.net Unit-2 Diverg Curl Problems - Mathematics -

Curl (mathematics)
Curl (mathematics) In vector calculus, the curl is a vector operator that describes the infinitesimal rotation of a 3-dimensional vector field.At every point in the field, the curl of that point is represented by a vector. -Video is targeted to blind users Attribution: Article text available under CC-BY-SA image source in video https://www.youtube.com/watch?v=nbDdQhreNDE -

ravish On physics of determinants and CURL Meaning of CURL.Mathematical physics
In this short presentation, the physical meaning of curl is explained .The curl representing the rotation of a vector is shown through diagrams. -

Curl of a vector field (ex. no.1): Vector Calculus
Free ebook http://tinyurl.com/EngMathYT I present and solve a simple example where the curl of a given vector field is sought. The curl is one of the basic operations of "vector calculus". Such and example is seen in 2nd year university mathematics. Such an example is seen in 2nd year university mathematics courses. -

-

OCN/GG312: Curl
OCN/GG312: Advanced Mathematics for Engineers and Scientists www.soest.hawaii.edu/GG/FACULTY/ITO/GG312 Prof. Garrett Apuzen-Ito University of Hawaii, Dept. of Geology & Geophysics, School of Ocean and Earth Science and Technology (SOEST) -

Math 392 Lecture 8 - Curl and Divergence and their interpretations
Welcome to lecture 8 of Vector Calculus and Linear Algebra! In this lecture, we start out by answering some homework problems on from section 13.4 of Stewart's Essential Calculus, ed 1. We then move on to section 13.5 where we discuss the curl and divergence of vector fields. We define curl and cover a few simple examples. Then I go on to state a very important theorem that ties together a lot of concepts that we've done--all the things that are equivalent to a vector field being conservative. Super important stuff. We move on to defining the divergence of a vector field, and give examples. Afterwards, we discuss the mathematical and physical interpretations of the curl and divergence of a vector field, complete with pictures! We end things there, and live to do math another day. -

Divergence of a vector field: Vector Calculus
Free ebook http://tinyurl.com/EngMathYT I present a simple example where I compute the divergence of a given vector field. I give a rough interpretation of the physical meaning of divergence. Such an example is seen in 2nd year university mathematics courses. -

Lecture 01: Beginning Algebra (Math 70)
Dr. James Curl of Modesto Junior College teaches beginning algebra. This first lesson reviews the course objectives and schedule, homework procedures, tests, grading, and similar protocols. The lecture covers numbers, why we use them, and some common formulas. Included are real numbers, integers, and the properties of real numbers (closure, commutative, associative, identity, inverse, distributive). -

Multivariable Calculus: Divergence & Curl in 3-Space
Watch more at http://educator.com/mathematics/multivariable-calculus/hovasapian/ Other subjects include Biology, Chemistry, Organic Chemistry, Statistics, Algebra 1/2, Geometry, Trigonometry, Calculus, Pre Calculus, Language, Music and Computer Science. -All lectures are broken down by individual topics -No more wasted time -Just search and jump directly to the answer -

Math 032 Multivariable Calculus 22 111914: Curl, Divergence, Green's Theorem
Del operator; curl of a vector field; physical interpretation of curl; irrotational fields; divergence of a vector field; physical interpretation of divergence; incompressible fields. Interpretation of Green's theorem (circulation-curl form); second form of Green's theorem (flux-divergence). -

"Divergence & Curl, Part 2" | Multivariable Calculus with Educator.com
"Divergence & Curl, Part 2" | Multivariable Calculus with Educator.com ►Watch more at http://educator.com/mathematics/multivariable-calculus/hovasapian/ Understand your Multivariable Calculus homework and ace the test with Educator.com's awesome hand-picked instructors. More features you'll see on Educator.com: -Full lessons complete with extra examples, downloads, and quizzes -Searchable and jumpable topics to save you time -Ability to ask questions to instructor and other students --- More subjects including: AP Physics B ► http://educator.com/physics/ap-physics-b/fullerton/ Linear Algebra ► http://educator.com/mathematics/linear-algebra/hovasapian/ Adobe Photoshop CS6 ► http://educator.com/software-training/adobe-photoshop-cs6/brown/ Our Multivariable Calculus Playlist ► http://www.youtube.com/playlist?list=PL552D5D5371A64405 --- http://Educator.com --- http://facebook.com/EducatorInc http://twitter.com/Educator http://youtube.com/EducatorVids -

"Divergence & Curl of a Vector Field" | Multivariable Calculus with Educator.com
"Divergence & Curl of a Vector Field" | Multivariable Calculus with Educator.com ►Watch more at http://educator.com/mathematics/multivariable-calculus/hovasapian/ Understand your Multivariable Calculus homework and ace the test with Educator.com's awesome hand-picked instructors. More features you'll see on Educator.com: -Full lessons complete with extra examples, downloads, and quizzes -Searchable and jumpable topics to save you time -Ability to ask questions to instructor and other students --- More subjects including: AP Physics B ► http://educator.com/physics/ap-physics-b/fullerton/ Linear Algebra ► http://educator.com/mathematics/linear-algebra/hovasapian/ Adobe Photoshop CS6 ► http://educator.com/software-training/adobe-photoshop-cs6/brown/ Our Multivariable Calculus Playlist ► http://www.youtube.com/playlist?list=PL552D5D5371A64405 --- http://Educator.com --- http://facebook.com/EducatorInc http://twitter.com/Educator http://youtube.com/EducatorVids
What is the Curl? Chris Tisdell UNSW
- Order: Reorder
- Duration: 48:35
- Updated: 12 May 2009
- views: 31024
- published: 12 May 2009
- views: 31024
Curl 1 | Partial derivatives, gradient, divergence, curl | Multivariable Calculus | Khan Academy
- Order: Reorder
- Duration: 9:33
- Updated: 12 Aug 2008
- views: 158401
- published: 12 Aug 2008
- views: 158401
Curl 3 | Partial derivatives, gradient, divergence, curl | Multivariable Calculus | Khan Academy
- Order: Reorder
- Duration: 10:23
- Updated: 12 Aug 2008
- views: 77750
- published: 12 Aug 2008
- views: 77750
Grad - Grad, Div and Curl (1/3)
- Order: Reorder
- Duration: 7:07
- Updated: 26 Jul 2011
- views: 42365
- published: 26 Jul 2011
- views: 42365
Div - Grad, Div and Curl (2/3)
- Order: Reorder
- Duration: 7:32
- Updated: 26 Jul 2011
- views: 47170
- published: 26 Jul 2011
- views: 47170
Curl - Grad, Div and Curl (3/3)
- Order: Reorder
- Duration: 10:28
- Updated: 26 Jul 2011
- views: 70205
- published: 26 Jul 2011
- views: 70205
UPenn Math 114 Introduction to how to Calculate Gradient, Divergence, and Curl
- Order: Reorder
- Duration: 20:15
- Updated: 14 Apr 2013
- views: 4915
- published: 14 Apr 2013
- views: 4915
GATE 2016- Engineering Mathematics- CURL of function Calculus part-10
- Order: Reorder
- Duration: 13:16
- Updated: 10 Oct 2015
- views: 1749
- published: 10 Oct 2015
- views: 1749
Divergence and Curl of a vector point function in Hindi
- Order: Reorder
- Duration: 56:03
- Updated: 08 Nov 2015
- views: 2883
- published: 08 Nov 2015
- views: 2883
GATE 2016- Engineering Mathematics- grad, curl, div, combined relations part-11
- Order: Reorder
- Duration: 5:12
- Updated: 11 Oct 2015
- views: 1798
- published: 11 Oct 2015
- views: 1798
Unit-2 Diverg Curl Problems - Mathematics
- Order: Reorder
- Duration: 28:02
- Updated: 04 Dec 2012
- views: 275
Curl (mathematics)
- Order: Reorder
- Duration: 14:52
- Updated: 22 Jan 2016
- views: 2
- published: 22 Jan 2016
- views: 2
ravish On physics of determinants and CURL Meaning of CURL.Mathematical physics
- Order: Reorder
- Duration: 0:58
- Updated: 31 Mar 2012
- views: 368
- published: 31 Mar 2012
- views: 368
Curl of a vector field (ex. no.1): Vector Calculus
- Order: Reorder
- Duration: 5:22
- Updated: 27 Mar 2009
- views: 21798
- published: 27 Mar 2009
- views: 21798
Curl of a vector field (ex. no.2): Vector calculus
- Order: Reorder
- Duration: 8:38
- Updated: 04 Apr 2009
- views: 14733
OCN/GG312: Curl
- Order: Reorder
- Duration: 29:47
- Updated: 20 Nov 2015
- views: 44
- published: 20 Nov 2015
- views: 44
Math 392 Lecture 8 - Curl and Divergence and their interpretations
- Order: Reorder
- Duration: 69:56
- Updated: 04 Apr 2013
- views: 4628
- published: 04 Apr 2013
- views: 4628
Divergence of a vector field: Vector Calculus
- Order: Reorder
- Duration: 6:21
- Updated: 05 Apr 2009
- views: 51611
Lecture 01: Beginning Algebra (Math 70)
- Order: Reorder
- Duration: 47:44
- Updated: 22 Jan 2013
- views: 17728
- published: 22 Jan 2013
- views: 17728
Multivariable Calculus: Divergence & Curl in 3-Space
- Order: Reorder
- Duration: 5:37
- Updated: 06 Jul 2012
- views: 212
- published: 06 Jul 2012
- views: 212
Math 032 Multivariable Calculus 22 111914: Curl, Divergence, Green's Theorem
- Order: Reorder
- Duration: 48:53
- Updated: 13 Oct 2015
- views: 14
- published: 13 Oct 2015
- views: 14
"Divergence & Curl, Part 2" | Multivariable Calculus with Educator.com
- Order: Reorder
- Duration: 9:27
- Updated: 06 Jul 2012
- views: 62
- published: 06 Jul 2012
- views: 62
"Divergence & Curl of a Vector Field" | Multivariable Calculus with Educator.com
- Order: Reorder
- Duration: 5:09
- Updated: 06 Jul 2012
- views: 629
- published: 06 Jul 2012
- views: 629
-

-

Calc III 15.1 Vector Fields and the Curl.avi
Introduction to vector fields, conservative fields, potential functions and the curl -

Tensor Calculus 1: The Rules of the Game
Textbook: http://bit.ly/ITCYTNew Solutions: http://bit.ly/ITACMS_Sol_Set_YT Errata: http://bit.ly/ITAErrata McConnell: http://bit.ly/MCTensors -

Tensor Calculus 3: The Covariant Basis
Textbook: http://bit.ly/ITCYTNew Solutions: http://bit.ly/ITACMS_Sol_Set_YT Errata: http://bit.ly/ITAErrata McConnell: http://bit.ly/MCTensors -

Tensor Calculus 0: Introduction
Textbook: http://bit.ly/ITCYTNew Solutions: http://bit.ly/ITACMS_Sol_Set_YT Errata: http://bit.ly/ITAErrata McConnell: http://bit.ly/MCTensors -

Concise Modular Calculus [95/97]: Curl & Stokes' Theorem (3/5 on Vector Calculus/Vector Analysis)
Explains how Stokes' Theorem is the mathematical manifestation of the observation that currents are surrounded by circular/cylindrical magnetic fields. Justifies, with special focus on fluid flow, electric fields and magnetic fields, how the curl is an indicator of vortices in a field. Concludes with a direct proof of Stokes' Theorem. All videos and slides for single variable calculus available at http://www2.latech.edu/~schroder/SVC_videos.htm. All videos and slides for multivariable calculus available at http://www2.latech.edu/~schroder/MVC_videos.htm. Public domain version of the electronic calculus textbook available at http://www.latech.edu/~schroder/SVC/Concise_Modular_Calculus.pdf. -

Calc III 2014 15.1 Vector Filelds and the Curl
Vector fields, conservative fields, the curl -

Divergence and Curl (Hindi) 1/2
-

-

VTU : Engineering Mathematics - I (Vector Calculus 1) Module 3 by SuperProf Chethan
SuperProfs - India’s largest online platform for exam preparation, brings you free access to online crash course of Engineering Mathematics - 1 according to syllabus prescribed by VTU. The complete course is free and covers detailed solution of highly expected questions for this semester. Salient Features of the course: - The course is FREE - Duration only 20 Hours - Focus on important topics - All 5 modules are covered - Detailed solution of expected questions - Taught by faculty with 15 years of experience -

Divergence & Curl in 3 Space, Multivariable Calcul CAM
Divergence & Curl in 3 Space, Multivariable Calcul CAM -

大学 講義 ベクトル解析(2005)L11 3次元ベクトル場の回転(rot,curl)
00:00:00 前回のQuiz解説(円柱座標での体積積分) 00:17:05 11 3次元のベクトル場の回転(rot) 00:17:05 11.1 渦無し条件の真実 00:28:02 -- ACM-ICPC国内予選に参戦報告 00:30:49 11.2 ベクトル場の回転(rot) 00:43:10 11.3 ベクトル場スカラー場のいろんな微分 00:51:32 --例題 勾配場には回転がないことの証明 01:01:58 11.4 ベクトル場スカラー場の微分での合成微分の利用 授業のページ http://www.a.math.ryukoku.ac.jp/~hig/course/vector_2005 演習問題 http://www.a.math.ryukoku.ac.jp/~hig/course/vector_2005/q11.pdf 解答 http://www.a.math.ryukoku.ac.jp/~hig/course/vector_2005/q11.pdf (アマチュア)スタッフによる手動撮影 龍谷大学大学教育開発センター2005年度FD自己応募研究プロジェクト"授業動画デジタルキャプチャ運用技術の開発と応用"の支援で撮影 -

Unit-2 Stoke's Theorem Problems - Mathematics
http://www.gurug.net Unit-2 Stoke's Theorem Problems - Mathematics -

James Clerk Maxwell: The Greatest Victorian Mathematical Physicists - Professor Raymond Flood
James Clerk Maxwell (1831-1879) was one of the most important mathematical physicists of all time, after only Newton and Einstein. Within a relatively short lifetime he made enormous contributions to science which this lecture will survey. Foremost among these was the formulation of the theory of electromagnetism with light, electricity and magnetism all shown to be manifestations of the electromagnetic field. He also made major contributions to the theory of colour vision and optics, the kinetic theory of gases and thermodynamics, and the understanding of the dynamics and stability of Saturn's rings. This talk was a part of the conference on '19th Century Mathematical Physics', held jointly by Gresham College and the British Society of the History of Mathematics. The transcript and downloadable versions of all of the lectures are available from the Gresham College website: http://www.gresham.ac.uk/lectures-and-events/19th-century-mathematical-physics Gresham College has been giving free public lectures since 1597. This tradition continues today with all of our five or so public lectures a week being made available for free download from our website. There is currently nearly 1,500 lectures free to access or download from the website. Website: http://www.gresham.ac.uk Twitter: http://twitter.com/GreshamCollege Facebook: http://www.facebook.com/pages/Gresham-College/14011689941 -

Lecture 23: Physical Mathematics at UNM Fall 2015
- Order: Reorder
- Duration: 69:03
- Updated: 07 Nov 2015
- views: 47
Calc III 15.1 Vector Fields and the Curl.avi
- Order: Reorder
- Duration: 59:19
- Updated: 12 Apr 2012
- views: 345
- published: 12 Apr 2012
- views: 345
Tensor Calculus 1: The Rules of the Game
- Order: Reorder
- Duration: 40:05
- Updated: 08 Feb 2014
- views: 43189
- published: 08 Feb 2014
- views: 43189
Tensor Calculus 3: The Covariant Basis
- Order: Reorder
- Duration: 37:01
- Updated: 14 Feb 2014
- views: 25790
- published: 14 Feb 2014
- views: 25790
Tensor Calculus 0: Introduction
- Order: Reorder
- Duration: 61:39
- Updated: 03 Feb 2014
- views: 99006
- published: 03 Feb 2014
- views: 99006
Concise Modular Calculus [95/97]: Curl & Stokes' Theorem (3/5 on Vector Calculus/Vector Analysis)
- Order: Reorder
- Duration: 62:14
- Updated: 26 Feb 2013
- views: 444
- published: 26 Feb 2013
- views: 444
Calc III 2014 15.1 Vector Filelds and the Curl
- Order: Reorder
- Duration: 56:02
- Updated: 10 Apr 2014
- views: 173
Divergence and Curl (Hindi) 1/2
- Order: Reorder
- Duration: 33:57
- Updated: 01 Aug 2015
- views: 1779
- published: 01 Aug 2015
- views: 1779
Divergence and Curl (Hindi)2/2
- Order: Reorder
- Duration: 29:41
- Updated: 03 Aug 2015
- views: 742
VTU : Engineering Mathematics - I (Vector Calculus 1) Module 3 by SuperProf Chethan
- Order: Reorder
- Duration: 60:56
- Updated: 28 Oct 2015
- views: 4346
- published: 28 Oct 2015
- views: 4346
Divergence & Curl in 3 Space, Multivariable Calcul CAM
- Order: Reorder
- Duration: 23:41
- Updated: 19 Jan 2015
- views: 24
大学 講義 ベクトル解析(2005)L11 3次元ベクトル場の回転(rot,curl)
- Order: Reorder
- Duration: 75:18
- Updated: 15 Nov 2013
- views: 1893
- published: 15 Nov 2013
- views: 1893
Unit-2 Stoke's Theorem Problems - Mathematics
- Order: Reorder
- Duration: 32:26
- Updated: 04 Dec 2012
- views: 6507
- published: 04 Dec 2012
- views: 6507
James Clerk Maxwell: The Greatest Victorian Mathematical Physicists - Professor Raymond Flood
- Order: Reorder
- Duration: 52:32
- Updated: 28 Nov 2012
- views: 188486
- published: 28 Nov 2012
- views: 188486
What is the curl?
- Order: Reorder
- Duration: 39:45
- Updated: 11 Aug 2011
- views: 6895
- published: 11 Aug 2011
- views: 6895
- Playlist
- Chat
- Playlist
- Chat

What is the Curl? Chris Tisdell UNSW
- Report rights infringement
- published: 12 May 2009
- views: 31024

Curl 1 | Partial derivatives, gradient, divergence, curl | Multivariable Calculus | Khan Academy
- Report rights infringement
- published: 12 Aug 2008
- views: 158401

Curl 3 | Partial derivatives, gradient, divergence, curl | Multivariable Calculus | Khan Academy
- Report rights infringement
- published: 12 Aug 2008
- views: 77750

Grad - Grad, Div and Curl (1/3)
- Report rights infringement
- published: 26 Jul 2011
- views: 42365

Div - Grad, Div and Curl (2/3)
- Report rights infringement
- published: 26 Jul 2011
- views: 47170

Curl - Grad, Div and Curl (3/3)
- Report rights infringement
- published: 26 Jul 2011
- views: 70205

UPenn Math 114 Introduction to how to Calculate Gradient, Divergence, and Curl
- Report rights infringement
- published: 14 Apr 2013
- views: 4915

GATE 2016- Engineering Mathematics- CURL of function Calculus part-10
- Report rights infringement
- published: 10 Oct 2015
- views: 1749

Divergence and Curl of a vector point function in Hindi
- Report rights infringement
- published: 08 Nov 2015
- views: 2883

GATE 2016- Engineering Mathematics- grad, curl, div, combined relations part-11
- Report rights infringement
- published: 11 Oct 2015
- views: 1798

Unit-2 Diverg Curl Problems - Mathematics
- Report rights infringement
- published: 04 Dec 2012
- views: 275

Curl (mathematics)
- Report rights infringement
- published: 22 Jan 2016
- views: 2

ravish On physics of determinants and CURL Meaning of CURL.Mathematical physics
- Report rights infringement
- published: 31 Mar 2012
- views: 368

Curl of a vector field (ex. no.1): Vector Calculus
- Report rights infringement
- published: 27 Mar 2009
- views: 21798
- Playlist
- Chat

Lecture 23: Physical Mathematics at UNM Fall 2015
- Report rights infringement
- published: 07 Nov 2015
- views: 47

Calc III 15.1 Vector Fields and the Curl.avi
- Report rights infringement
- published: 12 Apr 2012
- views: 345

Tensor Calculus 1: The Rules of the Game
- Report rights infringement
- published: 08 Feb 2014
- views: 43189

Tensor Calculus 3: The Covariant Basis
- Report rights infringement
- published: 14 Feb 2014
- views: 25790

Tensor Calculus 0: Introduction
- Report rights infringement
- published: 03 Feb 2014
- views: 99006

Concise Modular Calculus [95/97]: Curl & Stokes' Theorem (3/5 on Vector Calculus/Vector Analysis)
- Report rights infringement
- published: 26 Feb 2013
- views: 444

Calc III 2014 15.1 Vector Filelds and the Curl
- Report rights infringement
- published: 10 Apr 2014
- views: 173

Divergence and Curl (Hindi) 1/2
- Report rights infringement
- published: 01 Aug 2015
- views: 1779

Divergence and Curl (Hindi)2/2
- Report rights infringement
- published: 03 Aug 2015
- views: 742

VTU : Engineering Mathematics - I (Vector Calculus 1) Module 3 by SuperProf Chethan
- Report rights infringement
- published: 28 Oct 2015
- views: 4346

Divergence & Curl in 3 Space, Multivariable Calcul CAM
- Report rights infringement
- published: 19 Jan 2015
- views: 24

大学 講義 ベクトル解析(2005)L11 3次元ベクトル場の回転(rot,curl)
- Report rights infringement
- published: 15 Nov 2013
- views: 1893

Unit-2 Stoke's Theorem Problems - Mathematics
- Report rights infringement
- published: 04 Dec 2012
- views: 6507

James Clerk Maxwell: The Greatest Victorian Mathematical Physicists - Professor Raymond Flood
- Report rights infringement
- published: 28 Nov 2012
- views: 188486
[VIDEO]: Tens Of Thousands Visit Website Offering Alternative To U.S. Should Trump Win
Edit WorldNews.com 22 Feb 2016Prosecutor Says Uber Driver Admitted To Fatal Shooting Spree
Edit WorldNews.com 22 Feb 2016U.S., Russia Agree To Syrian Ceasefire At UN, Await Warring Parties To Sign Accord By Friday
Edit WorldNews.com 22 Feb 2016Laser-propelled spacecraft to reach Mars in 3 days?
Edit The Times of India 23 Feb 2016The Establishment vs. Bernie Sanders
Edit Huffington Post 22 Feb 2016Ashley Howard carries on family curling tradition
Edit Toronto Sun 23 Feb 2016Team Canada evens record while Alberta gets to 5-0 at Scotties (Canadian Curling Association)
Edit Public Technologies 23 Feb 2016Alberta wins squeaker to remain only unbeaten team at Scotties (Canadian Curling Association)
Edit Public Technologies 23 Feb 2016Kelly Capizzi (InterDigital Inc)
Edit Public Technologies 23 Feb 2016Indian Association for Blind celebrates annual day
Edit The Times of India 23 Feb 2016HeartChart – Feb. 21 Edition (Canadian Curling Association)
Edit Public Technologies 23 Feb 2016MANAGER: A game against a team in good form (Carlisle United FC Ltd)
Edit Public Technologies 23 Feb 2016Northern Ontario's Krista McCarville gets her curling groove back
Edit Canada Dot Com 23 Feb 2016New Educator Case Studies From Pearson Feature Improved Achievement in College and University Mathematics and ...
Edit Public Technologies 23 Feb 2016HeartChart – Feb. 20 Edition (Canadian Curling Association)
Edit Public Technologies 23 Feb 2016Pioneering DMU student is helping shape policy on STEM (De Montfort University)
Edit Public Technologies 23 Feb 2016Sundial Brands Enters Prestige Hair Category with Historic Launch of Madam C.J. Walker Beauty Culture Exclusively at Sephora - Iconic beauty brand's all-new haircare collection elevates maintenance, styling & treatment for all hair textures by fusing fair trade natural ingredients with performance innovation -- Continues Madam C.J. Walker's legacy of women's and economic empowerment -Greatness Begins with Confidence. POWERFUL. BEAUTIFUL. YOU.
Edit PR Newswire 23 Feb 2016Scope of agri-tourism discussed at meet
Edit The Times of India 23 Feb 2016- 1
- 2
- 3
- 4
- 5
- Next page »







