- published: 16 Jul 2015
- views: 277334
-
remove the playlistNet (polyhedron)
-
remove the playlistLatest Videos
-
remove the playlistLongest Videos
- remove the playlistNet (polyhedron)
- remove the playlistLatest Videos
- remove the playlistLongest Videos
- published: 10 Jul 2009
- views: 5923
- published: 16 Jul 2015
- views: 144288
- published: 13 Jun 2013
- views: 482
- published: 22 Jan 2016
- views: 32
- published: 25 Nov 2012
- views: 904
- published: 25 Jun 2013
- views: 60820
- published: 10 Jul 2009
- views: 245

In geometry the net of a polyhedron is an arrangement of edge-joined polygons in the plane which can be folded (along edges) to become the faces of the polyhedron. Polyhedral nets are a useful aid to the study of polyhedra and solid geometry in general, as they allow for models of polyhedra to be constructed from material such as thin cardboard.
For any given polyhedron that is unfolded into a net, many different equivalent nets can be formed depending on the choices of which edges are joined and which are separated.
It is a long-standing open question whether or not every convex polyhedron P (one without "dents" - in other words, all dihedral angles between the edges are ≤ 180 degrees) has a net: whether the surface P may be cut along edges and unfolded flat to a planar polygon (without overlap). (This system of edges is sometimes denoted as the polyhedron's unfolding.) The problem was first explicitly posed in a paper by Shephard. The history of and progress on this question is discussed in Part III of Geometric Folding Algorithms. If the restriction that the cuts be along polyhedron edges is relaxed to permit cuts through the interior of faces, then there are several known methods to cut and unfold a convex polyhedron to a planar polygon. For example, cutting along the cut locus of a point suffices.
This article is licensed under the Creative Commons Attribution-ShareAlike 3.0 Unported License, which means that you can copy and modify it as long as the entire work (including additions) remains under this license.
NET or Net may refer to:
In computing and communication:
In business and finance as an antonym of gross:
NET as an acronym may refer to:
In broadcast and media:
In science and psychology:
In mathematics:
In other uses:
.net or .NET may refer to:
This article is licensed under the Creative Commons Attribution-ShareAlike 3.0 Unported License, which means that you can copy and modify it as long as the entire work (including additions) remains under this license.
- Loading...

-
 4:19
4:19Finding surface area: nets of polyhedra | Perimeter, area, and volume | Geometry | Khan Academy
Finding surface area: nets of polyhedra | Perimeter, area, and volume | Geometry | Khan AcademyFinding surface area: nets of polyhedra | Perimeter, area, and volume | Geometry | Khan Academy
Now we shift our focus towards surface area (which is the sum of all the areas of all the shapes that cover the surface of the object). Let's apply it to the polyhedra net we learned about in the previous video. We'll walk you through it. Practice this lesson yourself on KhanAcademy.org right now: https://www.khanacademy.org/math/geometry/basic-geometry/volume_tutorial/e/surface-area?utm_source=YT&utm;_medium=Desc&utm;_campaign=Geometry Watch the next lesson: https://www.khanacademy.org/math/geometry/basic-geometry/volume_tutorial/v/solid-geometry-volume?utm_source=YT&utm;_medium=Desc&utm;_campaign=Geometry Missed the previous lesson? https://www.khanacademy.org/math/geometry/basic-geometry/volume_tutorial/v/nets-of-polyhedra?utm_source=YT&utm;_medium=Desc&utm;_campaign=Geometry Geometry on Khan Academy: We are surrounded by space. And that space contains lots of things. And these things have shapes. In geometry we are concerned with the nature of these shapes, how we define them, and what they teach us about the world at large--from math to architecture to biology to astronomy (and everything in between). Learning geometry is about more than just taking your medicine ("It's good for you!"), it's at the core of everything that exists--including you. Having said all that, some of the specific topics we'll cover include angles, intersecting lines, right triangles, perimeter, area, volume, circles, triangles, quadrilaterals, analytic geometry, and geometric constructions. Wow. That's a lot. To summarize: it's difficult to imagine any area of math that is more widely used than geometry. About Khan Academy: Khan Academy offers practice exercises, instructional videos, and a personalized learning dashboard that empower learners to study at their own pace in and outside of the classroom. We tackle math, science, computer programming, history, art history, economics, and more. Our math missions guide learners from kindergarten to calculus using state-of-the-art, adaptive technology that identifies strengths and learning gaps. We've also partnered with institutions like NASA, The Museum of Modern Art, The California Academy of Sciences, and MIT to offer specialized content. For free. For everyone. Forever. #YouCanLearnAnything Subscribe to Khan Academy’s Geometry channel: https://www.youtube.com/channel/UCD3OtKxPRUFw8kzYlhJXa1Q?sub_confirmation=1 Subscribe to Khan Academy: https://www.youtube.com/subscription_center?add_user=khanacademy -
 0:16
0:16Unfolding Polyhedron Nets
Unfolding Polyhedron NetsUnfolding Polyhedron Nets
http://demonstrations.wolfram.com/UnfoldingPolyhedronNets/ The Wolfram Demonstrations Project contains thousands of free interactive visualizations, with new entries added daily. A polyhedron can be unfolded from a series of connected faces in 3D to form a net of faces in a single plane. Two of the simplest polyhedra, the cube and tetrahedron, are presented here for interactive unfolding. Contributed by: Jon McLoone -
 6:48
6:48Nets of polyhedra | Perimeter, area, and volume | Geometry | Khan Academy
Nets of polyhedra | Perimeter, area, and volume | Geometry | Khan AcademyNets of polyhedra | Perimeter, area, and volume | Geometry | Khan Academy
We know...."nets of polyhedra" sounds like the title of a bad sci-fi movie about man-eating, muti-headed fish. A polyhedron ("polyhedra" is plural) is nothing more than a 3 dimensional shape with flat surfaces and straight edges (think: cube) Practice this lesson yourself on KhanAcademy.org right now: https://www.khanacademy.org/math/geometry/basic-geometry/volume_tutorial/e/nets-of-3d-figures?utm_source=YT&utm;_medium=Desc&utm;_campaign=Geometry Watch the next lesson: https://www.khanacademy.org/math/geometry/basic-geometry/volume_tutorial/v/surface-area-from-net?utm_source=YT&utm;_medium=Desc&utm;_campaign=Geometry Missed the previous lesson? https://www.khanacademy.org/math/geometry/basic-geometry/area_non_standard/v/perimeter-and-area-of-a-non-standard-polygon?utm_source=YT&utm;_medium=Desc&utm;_campaign=Geometry Geometry on Khan Academy: We are surrounded by space. And that space contains lots of things. And these things have shapes. In geometry we are concerned with the nature of these shapes, how we define them, and what they teach us about the world at large--from math to architecture to biology to astronomy (and everything in between). Learning geometry is about more than just taking your medicine ("It's good for you!"), it's at the core of everything that exists--including you. Having said all that, some of the specific topics we'll cover include angles, intersecting lines, right triangles, perimeter, area, volume, circles, triangles, quadrilaterals, analytic geometry, and geometric constructions. Wow. That's a lot. To summarize: it's difficult to imagine any area of math that is more widely used than geometry. About Khan Academy: Khan Academy offers practice exercises, instructional videos, and a personalized learning dashboard that empower learners to study at their own pace in and outside of the classroom. We tackle math, science, computer programming, history, art history, economics, and more. Our math missions guide learners from kindergarten to calculus using state-of-the-art, adaptive technology that identifies strengths and learning gaps. We've also partnered with institutions like NASA, The Museum of Modern Art, The California Academy of Sciences, and MIT to offer specialized content. For free. For everyone. Forever. #YouCanLearnAnything Subscribe to Khan Academy’s Geometry channel: https://www.youtube.com/channel/UCD3OtKxPRUFw8kzYlhJXa1Q?sub_confirmation=1 Subscribe to Khan Academy: https://www.youtube.com/subscription_center?add_user=khanacademy -
 4:23
4:23A unified net for folding polyhedron
A unified net for folding polyhedronA unified net for folding polyhedron
A Unified Net for Polyhedron Sequences This video clip demonstrates the power of one specific form of octahedral net in explaining a specific series of polyhedrons Comprising eight equilateral triangles, one of 11 known nets of the Octahedron, this octahedral net serves as the common denominator in a model that allows for the formation of many known polyhedral structures as well a many unknown formations. The octahedral net used here is powered by a magnetic pattern that controls the formation of all polyhedral structures derived from it. Based on these two findings, the model presented herein shows a new geometric sequence that allows for the transition from a two dimensional plane to the multidimensional plane of polyhedron solids by means of a continuous net comprising tessellated octahedron base unit nets. I believe these findings -- the specific octahedral net as well as the magnetic pattern controlling it -- are groundbreaking in the realm of mathematics and physics and seem to bear significance on our ability to understand structures in a multitude of other scientific field (C) All right reserved to tamir ashman (ISR) patent pending no 221671 (US) patent pending no 13/9756689 Permission is hereby granted to use these images for noncommercial purposes only. Inclusion in data collections, printed publications, commercial Web pages, or other forms of publication is prohibited. To obtain permission for commercial use or publication of these images please contact Tamir Ashman by mail www.platos-secret.com -
 4:00
4:00Net (polyhedron)
Net (polyhedron)Net (polyhedron)
Net (polyhedron) In geometry the net of a polyhedron is an arrangement of edge-joined polygons in the plane which can be folded (along edges) to become the faces of the polyhedron.Polyhedral nets are a useful aid to the study of polyhedra and solid geometry in general, as they allow for physical models of polyhedra to be constructed from material such as thin cardboard. =======Image-Copyright-Info======== License: Creative Commons Attribution-Share Alike 3.0 (CC BY-SA 3.0) LicenseLink: http://creativecommons.org/licenses/by-sa/3.0 Author-Info: Júlio Reis Image Source: https://en.wikipedia.org/wiki/File:Dodecahedron_flat.svg =======Image-Copyright-Info======== -Video is targeted to blind users Attribution: Article text available under CC-BY-SA image source in video https://www.youtube.com/watch?v=-52F4IBvaFo -
 1:50
1:50Origami Nets polyhedron with 8 triangular faces
Origami Nets polyhedron with 8 triangular facesOrigami Nets polyhedron with 8 triangular faces
What kind of polyhedron can be made from a net of 8 equilateral triangles. Surprisingly, two different solids can be made from the same net, depends on how you fold it. The net is suggested in http://mathworld.wolfram.com/Boat.html Origami Nets is a program downloaded from http://www.elica.net/site/museum/dalest/dalest.html -
 1:31
1:31Net of Solid Shapes - Icosahedron / Ікосаедр / Икосаэдр
Net of Solid Shapes - Icosahedron / Ікосаедр / ИкосаэдрNet of Solid Shapes - Icosahedron / Ікосаедр / Икосаэдр
Welcome to the ART KOSEKOMA Website http://kosekoma.com/ #icosahedron #ікосаедр #икосаэдр ------ Make a Three-Dimensional Figures and Their Nets with KOSEKOMA Делаем объемные фигуры и их развертки вместе с KOSEKOMA ------ Platonic solids: Icosahedron is a polyhedron with 20 faces. A regular icosahedron has 20 equilateral triangle faces with five meeting at each of its twelve vertices. Платоновые тела: Икосаэдр (от др.-греч. εἴκοσι «двадцать»; ἕδρον «сидение», «основание») — правильный выпуклый многогранник, двадцатигранник. Каждая из 20 граней представляет собой равносторонний треугольник. Число ребер равно 30, число вершин — 12. Икосаэдр имеет 59 звёздчатых форм. Платонові тіла: Ікосаедр (від грец. εικοσάς, «двадцять» і грец. —εδρον, «грань», «лице», «основа») — правильний опуклий багатогранник, двадцятигранник. Кожна з 20 граней є рівностороннім трикутником. Число ребер рівне 30, число вершин — 12. ----- ###0040###KOSEKOMA #nets #развертки #polyhedra #многогранники #MATHARTWORK #KOSEKOMA -
 0:08
0:08Two Convex Polyhedra with the Same Net
Two Convex Polyhedra with the Same NetTwo Convex Polyhedra with the Same Net
http://demonstrations.wolfram.com/TwoConvexPolyhedraWithTheSameNet/ The Wolfram Demonstrations Project contains thousands of free interactive visualizations, with new entries added daily. This Demonstration shows two convex polyhedra with the same net. Contributed by: Izidor Hafner -
 0:36
0:36Polyhedron using Conway transformation tttD
Polyhedron using Conway transformation tttD -
 0:57
0:57Polyhedron ammA8 created with Polyhedra for iPad/iPhone
Polyhedron ammA8 created with Polyhedra for iPad/iPhone
- Blender (software)
- Cardboard modeling
- Convex polyhedron
- Cube
- Cut locus
- Dihedral angles
- Dodecahedron
- Dymaxion map
- Eric W. Weisstein
- Erik Demaine
- Geometry
- George Olshevsky
- Higher dimensions
- Icosahedron
- Mathematica
- MathWorld
- Net (polyhedron)
- Paper model
- Polychoron
- Polygon
- Polyhedron
- Polytope
- Salvador Dalí
- Solid geometry
- Tesseract
- Triakis icosahedron
- Truncated tesseract
- UV mapping
- World map
-

Finding surface area: nets of polyhedra | Perimeter, area, and volume | Geometry | Khan Academy
Now we shift our focus towards surface area (which is the sum of all the areas of all the shapes that cover the surface of the object). Let's apply it to the polyhedra net we learned about in the previous video. We'll walk you through it. Practice this lesson yourself on KhanAcademy.org right now: https://www.khanacademy.org/math/geometry/basic-geometry/volume_tutorial/e/surface-area?utm_source=YT&utm;_medium=Desc&utm;_campaign=Geometry Watch the next lesson: https://www.khanacademy.org/math/geometry/basic-geometry/volume_tutorial/v/solid-geometry-volume?utm_source=YT&utm;_medium=Desc&utm;_campaign=Geometry Missed the previous lesson? https://www.khanacademy.org/math/geometry/basic-geometry/volume_tutorial/v/nets-of-polyhedra?utm_source=YT&utm;_medium=Desc&utm;_campaign=Geometry Geometry o... -

Unfolding Polyhedron Nets
http://demonstrations.wolfram.com/UnfoldingPolyhedronNets/ The Wolfram Demonstrations Project contains thousands of free interactive visualizations, with new entries added daily. A polyhedron can be unfolded from a series of connected faces in 3D to form a net of faces in a single plane. Two of the simplest polyhedra, the cube and tetrahedron, are presented here for interactive unfolding. Contributed by: Jon McLoone -

Nets of polyhedra | Perimeter, area, and volume | Geometry | Khan Academy
We know...."nets of polyhedra" sounds like the title of a bad sci-fi movie about man-eating, muti-headed fish. A polyhedron ("polyhedra" is plural) is nothing more than a 3 dimensional shape with flat surfaces and straight edges (think: cube) Practice this lesson yourself on KhanAcademy.org right now: https://www.khanacademy.org/math/geometry/basic-geometry/volume_tutorial/e/nets-of-3d-figures?utm_source=YT&utm;_medium=Desc&utm;_campaign=Geometry Watch the next lesson: https://www.khanacademy.org/math/geometry/basic-geometry/volume_tutorial/v/surface-area-from-net?utm_source=YT&utm;_medium=Desc&utm;_campaign=Geometry Missed the previous lesson? https://www.khanacademy.org/math/geometry/basic-geometry/area_non_standard/v/perimeter-and-area-of-a-non-standard-polygon?utm_source=YT&utm;_medium... -

A unified net for folding polyhedron
A Unified Net for Polyhedron Sequences This video clip demonstrates the power of one specific form of octahedral net in explaining a specific series of polyhedrons Comprising eight equilateral triangles, one of 11 known nets of the Octahedron, this octahedral net serves as the common denominator in a model that allows for the formation of many known polyhedral structures as well a many unknown formations. The octahedral net used here is powered by a magnetic pattern that controls the formation of all polyhedral structures derived from it. Based on these two findings, the model presented herein shows a new geometric sequence that allows for the transition from a two dimensional plane to the multidimensional plane of polyhedron solids by means of a continuous net comprising tessellated oct... -

Net (polyhedron)
Net (polyhedron) In geometry the net of a polyhedron is an arrangement of edge-joined polygons in the plane which can be folded (along edges) to become the faces of the polyhedron.Polyhedral nets are a useful aid to the study of polyhedra and solid geometry in general, as they allow for physical models of polyhedra to be constructed from material such as thin cardboard. =======Image-Copyright-Info======== License: Creative Commons Attribution-Share Alike 3.0 (CC BY-SA 3.0) LicenseLink: http://creativecommons.org/licenses/by-sa/3.0 Author-Info: Júlio Reis Image Source: https://en.wikipedia.org/wiki/File:Dodecahedron_flat.svg =======Image-Copyright-Info======== -Video is targeted to blind users Attribution: Article text available under CC-BY-SA image source in video https://www... -

Origami Nets polyhedron with 8 triangular faces
What kind of polyhedron can be made from a net of 8 equilateral triangles. Surprisingly, two different solids can be made from the same net, depends on how you fold it. The net is suggested in http://mathworld.wolfram.com/Boat.html Origami Nets is a program downloaded from http://www.elica.net/site/museum/dalest/dalest.html -

Net of Solid Shapes - Icosahedron / Ікосаедр / Икосаэдр
Welcome to the ART KOSEKOMA Website http://kosekoma.com/ #icosahedron #ікосаедр #икосаэдр ------ Make a Three-Dimensional Figures and Their Nets with KOSEKOMA Делаем объемные фигуры и их развертки вместе с KOSEKOMA ------ Platonic solids: Icosahedron is a polyhedron with 20 faces. A regular icosahedron has 20 equilateral triangle faces with five meeting at each of its twelve vertices. Платоновые тела: Икосаэдр (от др.-греч. εἴκοσι «двадцать»; ἕδρον «сидение», «основание») — правильный выпуклый многогранник, двадцатигранник. Каждая из 20 граней представляет собой равносторонний треугольник. Число ребер равно 30, число вершин — 12. Икосаэдр имеет 59 звёздчатых форм. Платонові тіла: Ікосаедр (від грец. εικοσάς, «двадцять» і грец. —εδρον, «грань», «лице», «основа») — правильний опуклий багато... -

Two Convex Polyhedra with the Same Net
http://demonstrations.wolfram.com/TwoConvexPolyhedraWithTheSameNet/ The Wolfram Demonstrations Project contains thousands of free interactive visualizations, with new entries added daily. This Demonstration shows two convex polyhedra with the same net. Contributed by: Izidor Hafner -

-

Finding surface area: nets of polyhedra | Perimeter, area, and volume | Geometry | Khan Academy
- Order: Reorder
- Duration: 4:19
- Updated: 16 Jul 2015
- views: 277334
- published: 16 Jul 2015
- views: 277334
Unfolding Polyhedron Nets
- Order: Reorder
- Duration: 0:16
- Updated: 10 Jul 2009
- views: 5923
- published: 10 Jul 2009
- views: 5923
Nets of polyhedra | Perimeter, area, and volume | Geometry | Khan Academy
- Order: Reorder
- Duration: 6:48
- Updated: 16 Jul 2015
- views: 144288
- published: 16 Jul 2015
- views: 144288
A unified net for folding polyhedron
- Order: Reorder
- Duration: 4:23
- Updated: 13 Jun 2013
- views: 482
- published: 13 Jun 2013
- views: 482
Net (polyhedron)
- Order: Reorder
- Duration: 4:00
- Updated: 22 Jan 2016
- views: 32
- published: 22 Jan 2016
- views: 32
Origami Nets polyhedron with 8 triangular faces
- Order: Reorder
- Duration: 1:50
- Updated: 25 Nov 2012
- views: 904
- published: 25 Nov 2012
- views: 904
Net of Solid Shapes - Icosahedron / Ікосаедр / Икосаэдр
- Order: Reorder
- Duration: 1:31
- Updated: 25 Jun 2013
- views: 60820
- published: 25 Jun 2013
- views: 60820
Two Convex Polyhedra with the Same Net
- Order: Reorder
- Duration: 0:08
- Updated: 10 Jul 2009
- views: 245
- published: 10 Jul 2009
- views: 245
Polyhedron using Conway transformation tttD
- Order: Reorder
- Duration: 0:36
- Updated: 28 Apr 2014
- views: 78
Polyhedron ammA8 created with Polyhedra for iPad/iPhone
- Order: Reorder
- Duration: 0:57
- Updated: 07 Jun 2015
- views: 25
-

Net of Truncated Triangular Trapezohedron / Розгортка усіченого трикутного трапецоедра
Welcome to the ART KOSEKOMA Website http://kosekoma.com/ ------ Truncated triangular trapezohedron (Dürer's solid). The truncated triangular trapezohedron is the first in an infinite series of truncated trapezohedron polyhedra. It has 6 pentagon and 2 triangle faces. This polyhedron can be constructed by truncating two opposite vertices of a cube, of a trigonal trapezohedron (a convex polyhedron with six congruent rhombus sides, formed by stretching or shrinking a cube along one of its long diagonals), or of a rhombohedron or parallelepiped (less symmetric polyhedra that still have the same combinatorial structure as a cube). In the case of a cube, or of a trigonal trapezohedron where the two truncated vertices are the ones on the stretching axes, the resulting shape has three-fold rotatio... -

-

-

Colored Szilassi Polyhedron
http://demonstrations.wolfram.com/ColoredSzilassiPolyhedron The Wolfram Demonstrations Project contains thousands of free interactive visualizations, with new entries added daily. This Demonstration shows a colored Szilassi polyhedron and its net. Contributed by: Izidor Hafner Audio created with WolframTones: http://tones.wolfram.com -

Mazes on the Edges of a Polyhedron
http://demonstrations.wolfram.com/MazesOnTheEdgesOfAPolyhedron The Wolfram Demonstrations Project contains thousands of free interactive visualizations, with new entries added daily. Find the shortest path from the light blue point to the orange point along the green edges of a net of a polyhedron. You can jump from one point on the net to another point that represents the same vertex of the polyhedron. Contributed by: Izidor Hafner Audio created with WolframTones: http://tones.wolfram.com -

Net of Truncated Triangular Trapezohedron / Розгортка усіченого трикутного трапецоедра
- Order: Reorder
- Duration: 1:25
- Updated: 27 Apr 2016
- views: 35
- published: 27 Apr 2016
- views: 35
LED Polyhedron
- Order: Reorder
- Duration: 1:23
- Updated: 11 Oct 2015
- views: 377
- published: 11 Oct 2015
- views: 377
Polyhedron using Conway transformation dkekD
- Order: Reorder
- Duration: 0:53
- Updated: 13 Jan 2015
- views: 63
Colored Szilassi Polyhedron
- Order: Reorder
- Duration: 0:49
- Updated: 27 Oct 2014
- views: 24
- published: 27 Oct 2014
- views: 24
Mazes on the Edges of a Polyhedron
- Order: Reorder
- Duration: 0:17
- Updated: 18 Jun 2014
- views: 24
- published: 18 Jun 2014
- views: 24
Polyhedron using Conway transformation (dd)tttD
- Order: Reorder
- Duration: 0:37
- Updated: 29 Apr 2014
- views: 51
-

SNUB POLYHEDRONS - 3DSOLID CREATION FROM APPROXIMATE GEOMETRIC CONSTRUCTION
This video shows creation of 3DSOLID entity - SNUB POLYHEDRON from approximate geometric construction... 3DSOLID has small SPHERES on its vertices as this operation was required to make possible building 3DSOLID entity using SURFSCULPT built-in AutoCAD command... More info and routines used to create such 3D model geometry you can find here : http://www.theswamp.org/index.php?topic=49862.0 (theswamp membership is required to access web page) Produced and constructed by Marko Ribar, d.i.a. (arch.) -

Geometry 2014 11.1 Polyhedrons and Cross Sections
Intro to polyhedrons, Euler's Formula and cross sections -

Ozric Tentacles - Technicians of the Sacred (2015, Full Album)
OT's 15th studio album. Purchase the album here http://www.amazon.com/Technicians-The-Sacred-Ozric-Tentacles/dp/B00V4UP28U https://www.burningshed.com/store/madfish/product/273/6519/ 00:00 The High Pass 08:23 Butterfly Garden 13:27 Far Memory 20:37 Changa Masala 26:41 Zingbong 35:07 Switchback 45:18 Epiphlioy 57:07 The Unusual Village 1:03:27 Smiling Potion 1:10:39 Rubbing Shoulders with the Absolute 1:19:15 Zenlike Creature -

-

Using Nets to find Surface Area
I walk students through using nets to find the surface area of four different polyhedrons. -

ORIGINS: Hieroglyphic Being | Resident Advisor
Subscribe to the RA YouTube channel to stay up to date: http://bit.ly/1x7PeGy Visit the RA feature page: http://www.residentadvisor.net/feature.aspx?2276 Club culture might play a large role in your life, but for most people partying is a pastime. For Jamal Moss, nightclubs are his saviour, his surrogate family and his creative fire. He grew up in Chicago in the 1980s amid complicated circumstances. Adoption, bullying, rebellion and, ultimately, homelessness led Moss to find solace in strange places. He was a regular at spots like Medusa's and the Reactor, where he began a musical and social education that continues to this day. House music became a gateway to other worlds and states of mind. These raw experiences eventually shaped Moss's music and DJing, as he made the sort of direct co... -

Class 15: General & Edge Unfolding
MIT 6.849 Geometric Folding Algorithms: Linkages, Origami, Polyhedra, Fall 2012 View the complete course: http://ocw.mit.edu/6-849F12 Instructor: Erik Demaine This class begins with defining handles and holes, and the Gauss-Bonnet Theorem applied to convex polyhedra. Algorithms for zipper unfolding, edge ununfoldable polyhedra, square-packing, band unfolding, and blooming of convex polyhedra are presented. License: Creative Commons BY-NC-SA More information at http://ocw.mit.edu/terms More courses at http://ocw.mit.edu -

Jardín de la Croix - Pomeroy (Full album) (HD)
Tracklist: 1. Polyhedron - 00:00 2. Jesse Harding - 08:55 3. Suomi - 15:03 4. Boston Steamer - 20:56 5. Antioquia - 30:22 6. Synaesthesia - 38:33 Recorded at Estudios Manitú (January 2008) Mixed at Estudios Manitú (January 2008) Mastered at Estudios Manitú (January 2008) Artwork by Alvaro Otero Released 01 March 2008 All songs written and recorded by Jardín de la Croix Ander Carballo/Guitar. Israel Arias/Drums Hugo Casqueiro/Bass. Pablo Rodríguez/Guitar - Free download here: http://jardindelacroix.bandcamp.com/album/pomeroy - Purchase the CD here: jardindelacroix@gmail.com -

古代汉语20_tienghoa.net
-

DIY Origami Ball - Christmas Star Ball - Ornament Stellated Icosahedron - Modular Origami
This tutorial will show you how to make an origami ball, or a Christmas star ball ornament. I love the stellated icosahedron shape, and like that it's a modular origami ball. Designer: Paolo Bascetta You can make these as home decorations for the holiday season. Spray them with glitter and hang them from strings for a homemade Christmas tree ornament. P.S. There is an error in the video. This model is actually the "Icosahedron," not "Dodecahedron." Thanks Jeremy Shafer for being a genius and pointing that out for me! *blushes* One of these days I'll get these shape names right! :-) ENJOY! :D Please subscribe for more craft tutorials to come! Make sure to check out my other origami tutorials at: http://www.youtube.com/playlist?list=PLUistU9g4l7RvOHS7TLbpYayJIfm3x7Ks FACEBOOK: https:/...
SNUB POLYHEDRONS - 3DSOLID CREATION FROM APPROXIMATE GEOMETRIC CONSTRUCTION
- Order: Reorder
- Duration: 25:44
- Updated: 30 Jul 2015
- views: 251
- published: 30 Jul 2015
- views: 251
Geometry 2014 11.1 Polyhedrons and Cross Sections
- Order: Reorder
- Duration: 31:04
- Updated: 28 Apr 2014
- views: 149
Ozric Tentacles - Technicians of the Sacred (2015, Full Album)
- Order: Reorder
- Duration: 89:21
- Updated: 05 Jun 2015
- views: 143384
- published: 05 Jun 2015
- views: 143384
Stellated Dodecahedron
- Order: Reorder
- Duration: 34:00
- Updated: 19 Mar 2014
- views: 9402
Using Nets to find Surface Area
- Order: Reorder
- Duration: 25:55
- Updated: 10 Feb 2014
- views: 100
- published: 10 Feb 2014
- views: 100
ORIGINS: Hieroglyphic Being | Resident Advisor
- Order: Reorder
- Duration: 20:34
- Updated: 10 Aug 2015
- views: 21851
- published: 10 Aug 2015
- views: 21851
Class 15: General & Edge Unfolding
- Order: Reorder
- Duration: 50:53
- Updated: 26 Aug 2014
- views: 291
- published: 26 Aug 2014
- views: 291
Jardín de la Croix - Pomeroy (Full album) (HD)
- Order: Reorder
- Duration: 42:39
- Updated: 22 Sep 2013
- views: 2172
- published: 22 Sep 2013
- views: 2172
古代汉语20_tienghoa.net
- Order: Reorder
- Duration: 45:47
- Updated: 21 Mar 2011
- views: 290
- published: 21 Mar 2011
- views: 290
DIY Origami Ball - Christmas Star Ball - Ornament Stellated Icosahedron - Modular Origami
- Order: Reorder
- Duration: 20:15
- Updated: 01 Nov 2013
- views: 20265
- published: 01 Nov 2013
- views: 20265
- Playlist
- Chat
- Playlist
- Chat

Finding surface area: nets of polyhedra | Perimeter, area, and volume | Geometry | Khan Academy
- Report rights infringement
- published: 16 Jul 2015
- views: 277334

Unfolding Polyhedron Nets
- Report rights infringement
- published: 10 Jul 2009
- views: 5923

Nets of polyhedra | Perimeter, area, and volume | Geometry | Khan Academy
- Report rights infringement
- published: 16 Jul 2015
- views: 144288

A unified net for folding polyhedron
- Report rights infringement
- published: 13 Jun 2013
- views: 482

Net (polyhedron)
- Report rights infringement
- published: 22 Jan 2016
- views: 32

Origami Nets polyhedron with 8 triangular faces
- Report rights infringement
- published: 25 Nov 2012
- views: 904

Net of Solid Shapes - Icosahedron / Ікосаедр / Икосаэдр
- Report rights infringement
- published: 25 Jun 2013
- views: 60820

Two Convex Polyhedra with the Same Net
- Report rights infringement
- published: 10 Jul 2009
- views: 245

Polyhedron using Conway transformation tttD
- Report rights infringement
- published: 28 Apr 2014
- views: 78

Polyhedron ammA8 created with Polyhedra for iPad/iPhone
- Report rights infringement
- published: 07 Jun 2015
- views: 25
- Playlist
- Chat

Net of Truncated Triangular Trapezohedron / Розгортка усіченого трикутного трапецоедра
- Report rights infringement
- published: 27 Apr 2016
- views: 35

LED Polyhedron
- Report rights infringement
- published: 11 Oct 2015
- views: 377

Polyhedron using Conway transformation dkekD
- Report rights infringement
- published: 13 Jan 2015
- views: 63

Colored Szilassi Polyhedron
- Report rights infringement
- published: 27 Oct 2014
- views: 24

Mazes on the Edges of a Polyhedron
- Report rights infringement
- published: 18 Jun 2014
- views: 24

Polyhedron using Conway transformation (dd)tttD
- Report rights infringement
- published: 29 Apr 2014
- views: 51
- Playlist
- Chat

SNUB POLYHEDRONS - 3DSOLID CREATION FROM APPROXIMATE GEOMETRIC CONSTRUCTION
- Report rights infringement
- published: 30 Jul 2015
- views: 251

Geometry 2014 11.1 Polyhedrons and Cross Sections
- Report rights infringement
- published: 28 Apr 2014
- views: 149

Ozric Tentacles - Technicians of the Sacred (2015, Full Album)
- Report rights infringement
- published: 05 Jun 2015
- views: 143384


Using Nets to find Surface Area
- Report rights infringement
- published: 10 Feb 2014
- views: 100

ORIGINS: Hieroglyphic Being | Resident Advisor
- Report rights infringement
- published: 10 Aug 2015
- views: 21851

Class 15: General & Edge Unfolding
- Report rights infringement
- published: 26 Aug 2014
- views: 291

Jardín de la Croix - Pomeroy (Full album) (HD)
- Report rights infringement
- published: 22 Sep 2013
- views: 2172

古代汉语20_tienghoa.net
- Report rights infringement
- published: 21 Mar 2011
- views: 290

DIY Origami Ball - Christmas Star Ball - Ornament Stellated Icosahedron - Modular Origami
- Report rights infringement
- published: 01 Nov 2013
- views: 20265
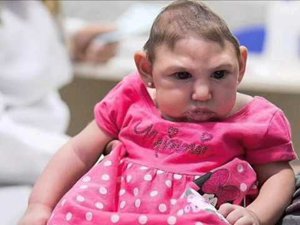
Baby with Zika-related microcephaly born at New Jersey hospital
Edit CNN 01 Jun 2016Iraq’s Green Zone Turns Red, Signals Phase Four Of Pacification
Edit WorldNews.com 01 Jun 2016Dozens of dead tiger cubs found in freezer at Thai temple
Edit The Irish Times 01 Jun 2016World's longest and deepest rail tunnel to open in Switzerland
Edit BBC News 01 Jun 2016Richest Self-Made Billionaires
Edit Forbes 01 Jun 2016Market Snapshot: Value of Canada’s net energy exports declined by over one-third in 2015 (National Energy Board)
Edit Public Technologies 01 Jun 2016Youngest Self-Made Women 2016
Edit Forbes 01 Jun 20161 June 2016 - UK investors favour fixed income and tracker funds in April (IMA - Investment Management Association)
Edit Public Technologies 01 Jun 2016G-III Apparel Group, Ltd. Announces First Quarter Fiscal 2017 Results (G-III Apparel Group Ltd)
Edit Public Technologies 01 Jun 2016Ghost net retrieved from northern Australian waters (AFMA - Australian Fisheries Management Authority)
Edit Public Technologies 01 Jun 2016Ghost net retrieved from northern Australian waters (ACS - Australian Customs and Border Protection Service)
Edit Public Technologies 01 Jun 2016Ghost net retrieved from northern Australian waters (Department of Immigration and Border Protection - Australian Government)
Edit Public Technologies 01 Jun 2016Net Asset Value(s) (Ecofin Water & Power Opportunities plc)
Edit Public Technologies 01 Jun 2016TRAI invites public response in new pre-consultation paper on Net Neutrality
Edit DNA India 01 Jun 2016Agree Realty Announces $79.5 Million Portfolio Acquisition (Agree Realty Corporation)
Edit Public Technologies 01 Jun 2016IOC, BPCL, HPCL among 10 stocks in focus today
Edit Yahoo Daily News 01 Jun 2016Making skateboards out of discarded fishing nets
Edit CNTV 01 Jun 2016- 1
- 2
- 3
- 4
- 5
- Next page »