- published: 11 Jun 2015
- views: 511739
-
remove the playlistLogarithm
- remove the playlistLogarithm
- published: 25 Oct 2011
- views: 179352
- published: 20 Aug 2012
- views: 276649
- published: 12 Sep 2010
- views: 334263
- published: 31 Oct 2015
- views: 4693
- published: 15 Nov 2007
- views: 740550

The logarithm of a number is the exponent by which another fixed value, the base, has to be raised to produce that number. For example, the logarithm of 1000 to base 10 is 3, because 1000 is 10 to the power 3: 1000 = 103 = 10 × 10 × 10. More generally, if x = by, then y is the logarithm of x to base b, and is written logb(x), so log10(1000) = 3.
Logarithms were introduced by John Napier in the early 17th century as a means to simplify calculations. They were rapidly adopted by scientists, engineers, and others to perform computations more easily, using slide rules and logarithm tables. These devices rely on the fact—important in its own right—that the logarithm of a product is the sum of the logarithms of the factors:
The present-day notion of logarithms comes from Leonhard Euler, who connected them to the exponential function in the 18th century.
The logarithm to base b = 10 is called the common logarithm and has many applications in science and engineering. The natural logarithm has the constant e (≈ 2.718) as its base; its use is widespread in pure mathematics, especially calculus. The binary logarithm uses base b = 2 and is prominent in computer science.
This article is licensed under the Creative Commons Attribution-ShareAlike 3.0 Unported License, which means that you can copy and modify it as long as the entire work (including additions) remains under this license.
- Loading...

-
 19:15
19:15❤² Logarithms... How? (mathbff)
❤² Logarithms... How? (mathbff)❤² Logarithms... How? (mathbff)
MIT grad introduces logs and shows how to evaluate them. To skip ahead: 1) For how to understand and evaluate BASIC LOGS, skip to time 0:52. 2) For how to evaluate weirder logs, including the log of 0, 1, a FRACTION, or a NEGATIVE number, skip to time 6:44. 3) For NATURAL LOGS (LN X), skip to time 11:17. 4) For even weirder logs, including SOLVING for X and using the CHANGE-OF-BASE formula, skip to time 14:56. Follow me on Twitter! http://twitter.com/mathbff 1) BASIC LOGS: you can read log notation as "log, base 3, of 9 equals X". The small (subscript) number is called the base. You can always evaluate a log expression by rearranging it into something called exponential form. Every log expression is connected to an exponential expression. In this example, the log is connected to the exponential form "3 to the X power equals 9". This means, "3 raised to what power gives you 9?" Since 3 raised to the power of 2 equals 9, the answer for X is 2. This is also the answer for the value of the log expression. The log is always equal to the power (or exponent) in the exponential version, and in this case it equals 2. If you want, you can find the log value in your head just by asking yourself what power you need in order to turn the base number into the middle number ("argument" number). Note: if there is no base number in the log expression (no little subscript number), then the base is 10, since 10 is the default base. 2) WEIRDER LOGS (log of 0, 1, a negative number, or a fraction): you can use the same steps to rearrange log expressions that have a fraction, negative number, 0, or 1 in them. You can still rearrange them to be in exponential form just like you can with the basic logs from earlier. The log of 1 will always be 0, since 0 is the only power that can turn a base into 1. The log of 0 will always be undefined, since no power can turn a base into 0. The log of a negative number is undefined in the real number system, since no real power can turn a positive base into a negative number. 3) NATURAL LOGS (ln x): the natural log is just a special type of log where the base is e (the special math constant e, which is approximately 2.718 if you plug it into your calculator). You can use the same steps for rearranging the log expression into exponential form. Just remember that ln x means log, base e. 4) EVEN WEIRDER LOGS (solving for X, change-of-base formula): even if there is an X variable in the log part of an equation, you can still rearrange the equation into exponential form. This will let you solve for X. Sometimes you might need to use the change-of-base formula to evaluate a log expression. If there is no whole number power you know that works, it may actually be a decimal power that you can find by using the change-of-base formula. For example, you can re-write log, base 2, of 7 as (log 7)/(log 2) and use your calculator to find the decimal number if you need it. For more of my math videos, check out: http://mathbff.com -
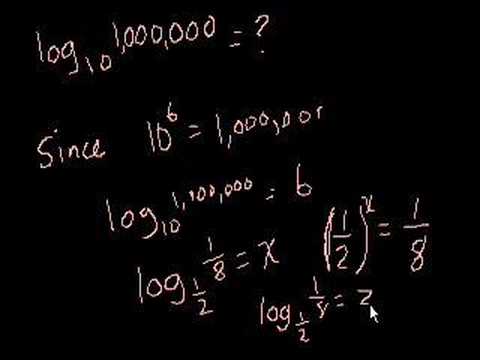 9:49
9:49Introduction to Logarithms
Introduction to LogarithmsIntroduction to Logarithms
An introduction to logarithms -
 4:28
4:28What is a Logarithm : Logarithms, Lesson 1
What is a Logarithm : Logarithms, Lesson 1 -
 14:26
14:26Logarithm Introduction
Logarithm IntroductionLogarithm Introduction
I introduce logarithms and show how to convert from logarithm form to exponential form. Yes, I know I mispelled logarithm on the chalk board. I blame it on the cold medicine I was taking! Here are my other PreCalculus lessons involving Logarithms http://www.profrobbob.com/pre-calculus/exponential-functions-and-logarithms Check out http://www.ProfRobBob.com, there you will find my lessons organized by class/subject and then by topics within each class. -
 3:34
3:34Logarithms, Explained - Steve Kelly
Logarithms, Explained - Steve KellyLogarithms, Explained - Steve Kelly
View full lesson: http://ed.ted.com/lessons/steve-kelly-logarithms-explained What are logarithms and why are they useful? Get the basics on these critical mathematical functions -- and discover why smart use of logarithms can determine whether your eyes turn red at the swimming pool this summer. Lesson by Steve Kelly, animation by TED-Ed. -
 3:08
3:08Solving Logarithmic Equations - Example 1
Solving Logarithmic Equations - Example 1Solving Logarithmic Equations - Example 1
Need a LIVE tutor to help answer a question? Check out the people at http://www.tutor.com/signup?AdDist=1&ExAdId;=90fddaff-89aa-4a4f-91da-90841e168246&TDC;_OfferCode=e2bf363b-0cb8-4635-98c3-2b5211d0847a&subject;=math&grade;=undefined for a FREE 30 minute session! Logarithm and Exponential Worksheet with Detailed Solutions made by me! : http://www.teacherspayteachers.com/Product/Algebra-Review-Exponential-and-Logarithm-Functions In this video, I solve a logarithmic equation using properties of logarithms and some other algebra techniques. -
 21:17
21:17Algebra 2 - Properties of Logarithms
Algebra 2 - Properties of Logarithms -
 80:17
80:17Logarithm by Gavesh Bharwaj (GB) Sir (ETOOSINDIA.COM)
Logarithm by Gavesh Bharwaj (GB) Sir (ETOOSINDIA.COM)Logarithm by Gavesh Bharwaj (GB) Sir (ETOOSINDIA.COM)
"Logarithm video Lecture of Maths for JEE Main and Advanced by Etoos india. GB Sir is known for his focused and simplified IIT-JEE teaching to bring to students an easy and analytical methodology towards IIT JEE. This course is designed and developed by the experienced faculty of KOTA and www.etoosindia.com. #maths gb logarithm m-gb-11-1301-logarithm-150413-vh" -
 9:16
9:16Introduction to logarithm properties | Logarithms | Algebra II | Khan Academy
Introduction to logarithm properties | Logarithms | Algebra II | Khan AcademyIntroduction to logarithm properties | Logarithms | Algebra II | Khan Academy
Introduction to the first two logarithm properties. Watch the next lesson: https://www.khanacademy.org/math/algebra2/logarithms-tutorial/logarithm_properties/v/introduction-to-logarithm-properties-part-2?utm_source=YT&utm;_medium=Desc&utm;_campaign=AlgebraII Missed the previous lesson? https://www.khanacademy.org/math/algebra2/logarithms-tutorial/logarithm_basics/v/graphing-logarithmic-functions?utm_source=YT&utm;_medium=Desc&utm;_campaign=AlgebraII Algebra II on Khan Academy: Your studies in algebra 1 have built a solid foundation from which you can explore linear equations, inequalities, and functions. In algebra 2 we build upon that foundation and not only extend our knowledge of algebra 1, but slowly become capable of tackling the BIG questions of the universe. We'll again touch on systems of equations, inequalities, and functions...but we'll also address exponential and logarithmic functions, logarithms, imaginary and complex numbers, conic sections, and matrices. Don't let these big words intimidate you. We're on this journey with you! About Khan Academy: Khan Academy offers practice exercises, instructional videos, and a personalized learning dashboard that empower learners to study at their own pace in and outside of the classroom. We tackle math, science, computer programming, history, art history, economics, and more. Our math missions guide learners from kindergarten to calculus using state-of-the-art, adaptive technology that identifies strengths and learning gaps. We've also partnered with institutions like NASA, The Museum of Modern Art, The California Academy of Sciences, and MIT to offer specialized content. For free. For everyone. Forever. #YouCanLearnAnything Subscribe to Khan Academy’s Algebra II channel: https://www.youtube.com/channel/UCsCA3_VozRtgUT7wWC1uZDg?sub_confirmation=1 Subscribe to Khan Academy: https://www.youtube.com/subscription_center?add_user=khanacademy -
 20:54
20:54Logarithm Part 1
Logarithm Part 1Logarithm Part 1
- Absolute value
- Absorbance
- Acid
- Acoustics
- Activity (chemistry)
- Addison-Wesley
- Algebraic geometry
- Algebraic number
- Algorithm
- Almost all
- Antiderivative
- Apparent magnitude
- Aqueous solution
- Arithmetic mean
- Arithmetic shift
- Astronomy
- Asymptotic
- B (musical note)
- Benford's law
- Big O notation
- Billiard table
- Binary logarithm
- Bit
- Boltzmann constant
- Branch cut
- B♭ (musical note)
- Calculus
- Carl Benjamin Boyer
- Carl Friedrich Gauss
- Celestial navigation
- Cent (music)
- Chain rule
- Chaos theory
- Chemistry
- Common logarithm
- Complex analysis
- Complex exponential
- Complex logarithm
- Complex number
- Complex numbers
- Complex plane
- Computer science
- Conjecture
- Continuous function
- Coordinate
- CORDIC
- Cosine
- CRC Press
- Cryptography
- Cube (algebra)
- Data set
- Decibel
- Decimal
- Decimal digit
- Degree (angle)
- Derivative
- Deterministic system
- Differential form
- Discrete logarithm
- Divergent sequence
- Donald Knuth
- Dover Publications
- Dynamical billiards
- Dynamical system
- Economics
- Edmund Wingate
- Engineering
- Entropy
- Equal temperament
- Equilateral triangle
- Erdős–Kac theorem
- Exponent
- Exponential function
- Exponential map
- Exponentiation
- Factorial
- Fair coin
- Fenske equation
- Finite field
- Finite groups
- Fitts's law
- Florian Cajori
- Forensic accounting
- Formula
- Fractal
- Fractal dimension
- Fractional part
- Frank W. J. Olver
- Frequency
- Geometric mean
- German gold mark
- German Papiermark
- Graph (mathematics)
- Graph of a function
- Group isomorphism
- Gunter's rule
- Haar measure
- Hausdorff dimension
- Hertz
- Hick's law
- Howard Eves
- Hue
- Human perception
- Hydrogen
- Hydronium
- Image compression
- Imaginary unit
- Increasing function
- Indian mathematics
- Infinity
- Information theory
- Integer
- Integer part
- Integral
- Interval (music)
- Inverse function
- Ion
- Irene Stegun
- Irrational number
- Irving Stringham
- ISO 31-11
- Iterated logarithm
- IUPAC
- Johannes Kepler
- John Napier
- John Wiley & Sons
- Joost Bürgi
- Just major third
- Jürgen Neukirch
- Keith Devlin
- Lambert W function
- Law of large numbers
- Lebesgue measure
- Leonhard Euler
- Likelihood function
- Limit (mathematics)
- Limit of a sequence
- Log-log
- Logarithm
- Logarithm table
- Logarithmic growth
- Logarithmic scale
- Logarithmic spiral
- Logistic function
- Logit
- Logos
- Lyapunov exponent
- Lynn Nadel
- Major third
- Mathematical Reviews
- Mathematical table
- Matrix exponential
- McGraw-Hill
- Memory (computing)
- Merge sort
- Michael Stifel
- Milton Abramowitz
- Molar concentration
- Monotonic function
- Morris Kline
- Natural logarithm
- Natural number
- Nautilus
- Nernst equation
- Newton's method
- Nicholas Higham
- Nicholas Mercator
- Nicolas Bourbaki
- Noise (electronic)
- Normal distribution
- Nth root
- Number theory
- Nuremberg
- O'Reilly Media
- Octave
- Optics
- Origin (mathematics)
- Parametric model
- Paul Halmos
- PH
- Pi
- Pierre-Simon Laplace
- Pitch (music)
- Polar form
- Polylogarithm
- Positive number
- Power (physics)
- Power law
- Power series
- Prime factor
- Prime number
- Prime number theorem
- Probability theory
- Programming language
- Prosthaphaeresis
- Psychophysics
- PubMed Central
- PubMed Identifier
- Pure mathematics
- Quicksort
- Radian
- Radix
- Random variable
- Ratio
- Rational number
- Real number
- Recursion
- Reflection (physics)
- Richter scale
- Riemann hypothesis
- Rolf Nevanlinna
- Scale invariance
- Self-similarity
- Semilog
- Semitone
- Serge Lang
- Series (mathematics)
- Sierpinski triangle
- Sigma notation
- Significand
- Sine
- Slide rule
- Slope
- Sound pressure
- Spectrometer
- Springer-Verlag
- Square root of 2
- Statistical model
- Statistics
- Stevens' power law
- Stirling's formula
- Sum
- Super-logarithm
- Surveying
- Tangent
- Tangent space
- Taylor series
- Tetration
- Time complexity
- Tritone
- Turn (geometry)
- Umberto Eco
- Uniform cost model
- Virasena
- Voltage
- Weber–Fechner law
- Wikipedia Media help
- William Kahan
- William Oughtred
- Wolfram Research
- World Scientific
- X axis
- Zech's logarithm
- Carl Benjamin Boyer
- Carl Friedrich Gauss
- Donald Knuth
- Edmund Wingate
- Florian Cajori
- Howard Eves
- Irene Stegun
- Irving Stringham
- Johannes Kepler
- John Napier
- Jürgen Neukirch
- Keith Devlin
- Leonhard Euler
- Lynn Nadel
- Michael Stifel
- Milton Abramowitz
- Morris Kline
- Nicholas Higham
- Nicholas Mercator
- Paul Halmos
- Rolf Nevanlinna
- Serge Lang
- Umberto Eco
- William Kahan
- William Oughtred
-

❤² Logarithms... How? (mathbff)
MIT grad introduces logs and shows how to evaluate them. To skip ahead: 1) For how to understand and evaluate BASIC LOGS, skip to time 0:52. 2) For how to evaluate weirder logs, including the log of 0, 1, a FRACTION, or a NEGATIVE number, skip to time 6:44. 3) For NATURAL LOGS (LN X), skip to time 11:17. 4) For even weirder logs, including SOLVING for X and using the CHANGE-OF-BASE formula, skip to time 14:56. Follow me on Twitter! http://twitter.com/mathbff 1) BASIC LOGS: you can read log notation as "log, base 3, of 9 equals X". The small (subscript) number is called the base. You can always evaluate a log expression by rearranging it into something called exponential form. Every log expression is connected to an exponential expression. In this example, the log is connected to the expo... -
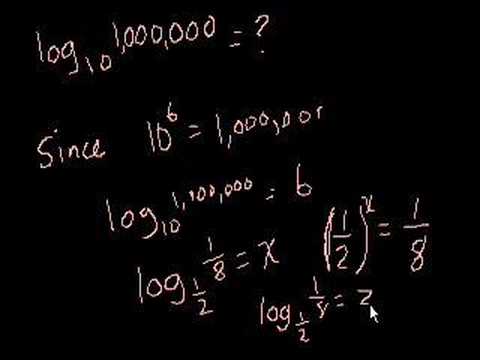
Introduction to Logarithms
An introduction to logarithms -

-

Logarithm Introduction
I introduce logarithms and show how to convert from logarithm form to exponential form. Yes, I know I mispelled logarithm on the chalk board. I blame it on the cold medicine I was taking! Here are my other PreCalculus lessons involving Logarithms http://www.profrobbob.com/pre-calculus/exponential-functions-and-logarithms Check out http://www.ProfRobBob.com, there you will find my lessons organized by class/subject and then by topics within each class. -

Logarithms, Explained - Steve Kelly
View full lesson: http://ed.ted.com/lessons/steve-kelly-logarithms-explained What are logarithms and why are they useful? Get the basics on these critical mathematical functions -- and discover why smart use of logarithms can determine whether your eyes turn red at the swimming pool this summer. Lesson by Steve Kelly, animation by TED-Ed. -

Solving Logarithmic Equations - Example 1
Need a LIVE tutor to help answer a question? Check out the people at http://www.tutor.com/signup?AdDist=1&ExAdId;=90fddaff-89aa-4a4f-91da-90841e168246&TDC;_OfferCode=e2bf363b-0cb8-4635-98c3-2b5211d0847a&subject;=math&grade;=undefined for a FREE 30 minute session! Logarithm and Exponential Worksheet with Detailed Solutions made by me! : http://www.teacherspayteachers.com/Product/Algebra-Review-Exponential-and-Logarithm-Functions In this video, I solve a logarithmic equation using properties of logarithms and some other algebra techniques. -

-

Logarithm by Gavesh Bharwaj (GB) Sir (ETOOSINDIA.COM)
"Logarithm video Lecture of Maths for JEE Main and Advanced by Etoos india. GB Sir is known for his focused and simplified IIT-JEE teaching to bring to students an easy and analytical methodology towards IIT JEE. This course is designed and developed by the experienced faculty of KOTA and www.etoosindia.com. #maths gb logarithm m-gb-11-1301-logarithm-150413-vh" -

Introduction to logarithm properties | Logarithms | Algebra II | Khan Academy
Introduction to the first two logarithm properties. Watch the next lesson: https://www.khanacademy.org/math/algebra2/logarithms-tutorial/logarithm_properties/v/introduction-to-logarithm-properties-part-2?utm_source=YT&utm;_medium=Desc&utm;_campaign=AlgebraII Missed the previous lesson? https://www.khanacademy.org/math/algebra2/logarithms-tutorial/logarithm_basics/v/graphing-logarithmic-functions?utm_source=YT&utm;_medium=Desc&utm;_campaign=AlgebraII Algebra II on Khan Academy: Your studies in algebra 1 have built a solid foundation from which you can explore linear equations, inequalities, and functions. In algebra 2 we build upon that foundation and not only extend our knowledge of algebra 1, but slowly become capable of tackling the BIG questions of the universe. We'll again touch on sys... -

Logarithm Part 1
❤² Logarithms... How? (mathbff)
- Order: Reorder
- Duration: 19:15
- Updated: 11 Jun 2015
- views: 511739
- published: 11 Jun 2015
- views: 511739
Introduction to Logarithms
- Order: Reorder
- Duration: 9:49
- Updated: 17 May 2007
- views: 1005893
- published: 17 May 2007
- views: 1005893
What is a Logarithm : Logarithms, Lesson 1
- Order: Reorder
- Duration: 4:28
- Updated: 31 Aug 2012
- views: 191563
Logarithm Introduction
- Order: Reorder
- Duration: 14:26
- Updated: 25 Oct 2011
- views: 179352
- published: 25 Oct 2011
- views: 179352
Logarithms, Explained - Steve Kelly
- Order: Reorder
- Duration: 3:34
- Updated: 20 Aug 2012
- views: 276649
- published: 20 Aug 2012
- views: 276649
Solving Logarithmic Equations - Example 1
- Order: Reorder
- Duration: 3:08
- Updated: 12 Sep 2010
- views: 334263
- published: 12 Sep 2010
- views: 334263
Algebra 2 - Properties of Logarithms
- Order: Reorder
- Duration: 21:17
- Updated: 26 Feb 2013
- views: 223416
Logarithm by Gavesh Bharwaj (GB) Sir (ETOOSINDIA.COM)
- Order: Reorder
- Duration: 80:17
- Updated: 31 Oct 2015
- views: 4693
- published: 31 Oct 2015
- views: 4693
Introduction to logarithm properties | Logarithms | Algebra II | Khan Academy
- Order: Reorder
- Duration: 9:16
- Updated: 15 Nov 2007
- views: 740550
- published: 15 Nov 2007
- views: 740550
Logarithm Part 1
- Order: Reorder
- Duration: 20:54
- Updated: 14 Nov 2013
- views: 10218
- published: 14 Nov 2013
- views: 10218
- Playlist
- Chat
- Playlist
- Chat

❤² Logarithms... How? (mathbff)
- Report rights infringement
- published: 11 Jun 2015
- views: 511739
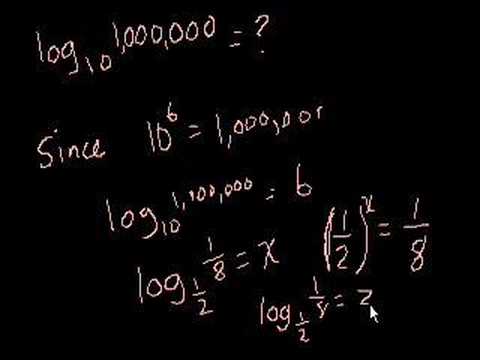
Introduction to Logarithms
- Report rights infringement
- published: 17 May 2007
- views: 1005893

What is a Logarithm : Logarithms, Lesson 1
- Report rights infringement
- published: 31 Aug 2012
- views: 191563

Logarithm Introduction
- Report rights infringement
- published: 25 Oct 2011
- views: 179352

Logarithms, Explained - Steve Kelly
- Report rights infringement
- published: 20 Aug 2012
- views: 276649

Solving Logarithmic Equations - Example 1
- Report rights infringement
- published: 12 Sep 2010
- views: 334263

Algebra 2 - Properties of Logarithms
- Report rights infringement
- published: 26 Feb 2013
- views: 223416

Logarithm by Gavesh Bharwaj (GB) Sir (ETOOSINDIA.COM)
- Report rights infringement
- published: 31 Oct 2015
- views: 4693

Introduction to logarithm properties | Logarithms | Algebra II | Khan Academy
- Report rights infringement
- published: 15 Nov 2007
- views: 740550

Logarithm Part 1
- Report rights infringement
- published: 14 Nov 2013
- views: 10218
Brexit and the disunited Kingdom
Edit Jamaica Observer 03 Jul 2016City deaf to crying need for sound barriers
Edit The Times of India 30 Jun 2016Sound attenuation at shooting ranges
Edit The Examiner 26 Jun 2016The Secret Group's Secret Weapon? Party King Andrew W.K.
Edit Houston Press 22 Jun 2016Review: 'Central Intelligence' squanders Kevin Hart and Dwayne Johnson's comic chemistry
Edit Syracuse 19 Jun 2016We’ve Almost Reached Peak Fossil Fuels for Electricity
Edit Bloomberg 13 Jun 2016Climate change could trigger tropical evacuations, researchers advise (University of California - Berkeley)
Edit Public Technologies 09 Jun 2016Relevance of in vitro biophysical studies [Biological Sciences]>
Edit PNAS 07 Jun 2016The Women Behind the Jet Propulsion Laboratory
Edit The Atlantic 01 Jun 2016Relevance of in vitro biophysical studies [Letters (Online Only)]>
Edit PNAS 25 May 2016LexisNexis Introduces New, Interactive Rule of Law Awareness Tool (Reed Elsevier plc)
Edit Public Technologies 24 May 2016LexisNexis Introduces New, Interactive Rule of Law Awareness Tool (LexisNexis Group)
Edit Public Technologies 24 May 2016Methodology behind the Guardian University Guide 2017
Edit The Guardian 23 May 2016PhD aspirants allege discrepancies in examination process
Edit Indian Express 16 May 2016- 1
- 2
- 3
- 4
- 5
- Next page »



