-
Convergence and Divergence - Introduction to Series
This calculus 2 video tutorial provides a basic introduction into series. It explains how to determine the convergence and divergence of a series. It explains the difference between a sequence and a series. This video includes examples and practice problems with geometric series, harmonic series, and the telescoping series. It also discusses the divergence test.
Get The Full 50 Minute Video on Patreon:
https://www.patreon.com/MathScienceTutor
Direct Link to The Full Video:
https://bit.ly/3BR6IPm
Full 50 Minute Video on Youtube:
https://www.youtube.com/watch?v=Bz8N2qcghBQ
Join The Membership Program:
https://www.youtube.com/channel/UCEWpbFLzoYGPfuWUMFPSaoA/join
published: 20 Jan 2021
-
Convergence and Divergence: The Return of Sequences and Series
We learned a little bit about sequences and series earlier in the mathematics course, but now its time to work with these some more, now that we understand calculus! First up, what does it mean for a sequence or series to be convergent or divergent, and how can we tell which one it is? Let's find out!
Watch the whole Calculus playlist: http://bit.ly/ProfDaveCalculus
Watch the whole Mathematics playlist: http://bit.ly/ProfDaveMath
Classical Physics Tutorials: http://bit.ly/ProfDavePhysics1
Modern Physics Tutorials: http://bit.ly/ProfDavePhysics2
General Chemistry Tutorials: http://bit.ly/ProfDaveGenChem
Organic Chemistry Tutorials: http://bit.ly/ProfDaveOrgChem
Biochemistry Tutorials: http://bit.ly/ProfDaveBiochem
Biology Tutorials: http://bit.ly/ProfDaveBio
EMAIL► ProfessorDaveExplains@...
published: 15 Jun 2018
-
The Coming Convergence Full Movie
"The Coming Convergence" 2017
published: 01 Feb 2020
-
Convergence
Provided to YouTube by Beggars Group Digital Ltd.
Convergence · Jonny Greenwood
Bodysong.
℗ 2003 XL Recordings Ltd
Released on: 2003-10-27
Engineer: Christian Wright
Engineer: Graeme Stewart
Producer: Graeme Stewart
Producer: Jonny Greenwood
Associated Performer: Jonny Greenwood
Engineer: Simon Gibson
Music Publisher: Warner Chappell Music Publishing Ltd.
Music Publisher: Harry Fox Agency
Music Publisher: CMRRA
Composer Lyricist: Jonny Greenwood
Auto-generated by YouTube.
published: 02 Jan 2020
-
Convergent and divergent sequences | Series | AP Calculus BC | Khan Academy
Courses on Khan Academy are always 100% free. Start practicing—and saving your progress—now: https://www.khanacademy.org/math/ap-calculus-bc/bc-series-new/bc-10-1/v/convergent-and-divergent-sequences
Using the recursive formula of a sequence to find its fifth term. Created by Sal Khan.
Practice this lesson yourself on KhanAcademy.org right now: https://www.khanacademy.org/math/ap-calculus-bc/bc-series/bc-inf-sequences/e/convergence-and-divergence-of-sequences?utm_source=YT&utm;_medium=Desc&utm;_campaign=APCalculusBC
Watch the next lesson: https://www.khanacademy.org/math/ap-calculus-bc/bc-series/bc-inf-sequences/v/identifying-sequence-convergence-divergence?utm_source=YT&utm;_medium=Desc&utm;_campaign=APCalculusBC
Missed the previous lesson? https://www.khanacademy.org/math/ap-calculus-b...
published: 15 Feb 2013
-
Convergence: Courage in a Crisis | Official Trailer | Netflix
An epic collaboration that spans eight countries and nine individual stories, Convergence reveals the power of compassion and community in the face of a crisis. Only on Netflix October 12.
Featuring the original song "Beautiful Life" from Michael Kiwanuka.
SUBSCRIBE: http://bit.ly/29qBUt7
About Netflix:
Netflix is the world's leading streaming entertainment service with over 209 million paid memberships in over 190 countries enjoying TV series, documentaries and feature films across a wide variety of genres and languages. Members can watch as much as they want, anytime, anywhere, on any internet-connected screen. Members can play, pause and resume watching, all without commercials or commitments.
Convergence: Courage in a Crisis | Official Trailer | Netflix
https://youtube.com/Netfli...
published: 27 Sep 2021
-
Convergence
Provided to YouTube by Base79
Convergence · Random Factor
Convergence
℗ 2020Vision
Released on: 2004-01-01
Auto-generated by YouTube.
published: 10 Jun 2016
-
Infinite Series(Part-I): Convergence in hindi
This video is useful for students of BSc/MSc Mathematics students. Also for students preparing IIT-JAM, GATE, CSIR-NET and other exams.
उम्मीद हे आपको ये videos पसंद आ रही होगी, यदि कोई भी doubt या Problem हो तो शुरआत में आप callme4 app की ID "gr8bhagwan@cm4" पर फ़ोन कर सकते है. और पसंद वाली वीडियो को Like, Shear और सब्सक्राइब (Subscribe) करना न भूले, इससे क्रिएटर (Creator) को और ज़्यादा काम करने की प्रेरणा मिलती हे
keep support us to create "Skilled & educated India" we request you to visit & subscribe our Associated Channels to give us more strength.
#01 MotorLAB Ev's (For Electrical)
https://www.youtube.com/c/MotorLabEvs...
#02 Dailyinnovation4life (For Civil Engg.)
https://www.youtube.com/channel/UC75l...
#03 Vedam Compititive classes
https://www.youtube.com/channel/UCASK...
#04 Gr8...
published: 04 Sep 2016
16:18
Convergence and Divergence - Introduction to Series
This calculus 2 video tutorial provides a basic introduction into series. It explains how to determine the convergence and divergence of a series. It explains...
This calculus 2 video tutorial provides a basic introduction into series. It explains how to determine the convergence and divergence of a series. It explains the difference between a sequence and a series. This video includes examples and practice problems with geometric series, harmonic series, and the telescoping series. It also discusses the divergence test.
Get The Full 50 Minute Video on Patreon:
https://www.patreon.com/MathScienceTutor
Direct Link to The Full Video:
https://bit.ly/3BR6IPm
Full 50 Minute Video on Youtube:
https://www.youtube.com/watch?v=Bz8N2qcghBQ
Join The Membership Program:
https://www.youtube.com/channel/UCEWpbFLzoYGPfuWUMFPSaoA/join
https://wn.com/Convergence_And_Divergence_Introduction_To_Series
This calculus 2 video tutorial provides a basic introduction into series. It explains how to determine the convergence and divergence of a series. It explains the difference between a sequence and a series. This video includes examples and practice problems with geometric series, harmonic series, and the telescoping series. It also discusses the divergence test.
Get The Full 50 Minute Video on Patreon:
https://www.patreon.com/MathScienceTutor
Direct Link to The Full Video:
https://bit.ly/3BR6IPm
Full 50 Minute Video on Youtube:
https://www.youtube.com/watch?v=Bz8N2qcghBQ
Join The Membership Program:
https://www.youtube.com/channel/UCEWpbFLzoYGPfuWUMFPSaoA/join
- published: 20 Jan 2021
- views: 1326479
9:40
Convergence and Divergence: The Return of Sequences and Series
We learned a little bit about sequences and series earlier in the mathematics course, but now its time to work with these some more, now that we understand calc...
We learned a little bit about sequences and series earlier in the mathematics course, but now its time to work with these some more, now that we understand calculus! First up, what does it mean for a sequence or series to be convergent or divergent, and how can we tell which one it is? Let's find out!
Watch the whole Calculus playlist: http://bit.ly/ProfDaveCalculus
Watch the whole Mathematics playlist: http://bit.ly/ProfDaveMath
Classical Physics Tutorials: http://bit.ly/ProfDavePhysics1
Modern Physics Tutorials: http://bit.ly/ProfDavePhysics2
General Chemistry Tutorials: http://bit.ly/ProfDaveGenChem
Organic Chemistry Tutorials: http://bit.ly/ProfDaveOrgChem
Biochemistry Tutorials: http://bit.ly/ProfDaveBiochem
Biology Tutorials: http://bit.ly/ProfDaveBio
EMAIL► ProfessorDaveExplains@gmail.com
PATREON► http://patreon.com/ProfessorDaveExplains
Check out "Is This Wi-Fi Organic?", my book on disarming pseudoscience!
Amazon: https://amzn.to/2HtNpVH
Bookshop: https://bit.ly/39cKADM
Barnes and Noble: https://bit.ly/3pUjmrn
Book Depository: http://bit.ly/3aOVDlT
https://wn.com/Convergence_And_Divergence_The_Return_Of_Sequences_And_Series
We learned a little bit about sequences and series earlier in the mathematics course, but now its time to work with these some more, now that we understand calculus! First up, what does it mean for a sequence or series to be convergent or divergent, and how can we tell which one it is? Let's find out!
Watch the whole Calculus playlist: http://bit.ly/ProfDaveCalculus
Watch the whole Mathematics playlist: http://bit.ly/ProfDaveMath
Classical Physics Tutorials: http://bit.ly/ProfDavePhysics1
Modern Physics Tutorials: http://bit.ly/ProfDavePhysics2
General Chemistry Tutorials: http://bit.ly/ProfDaveGenChem
Organic Chemistry Tutorials: http://bit.ly/ProfDaveOrgChem
Biochemistry Tutorials: http://bit.ly/ProfDaveBiochem
Biology Tutorials: http://bit.ly/ProfDaveBio
EMAIL► ProfessorDaveExplains@gmail.com
PATREON► http://patreon.com/ProfessorDaveExplains
Check out "Is This Wi-Fi Organic?", my book on disarming pseudoscience!
Amazon: https://amzn.to/2HtNpVH
Bookshop: https://bit.ly/39cKADM
Barnes and Noble: https://bit.ly/3pUjmrn
Book Depository: http://bit.ly/3aOVDlT
- published: 15 Jun 2018
- views: 325104
4:27
Convergence
Provided to YouTube by Beggars Group Digital Ltd.
Convergence · Jonny Greenwood
Bodysong.
℗ 2003 XL Recordings Ltd
Released on: 2003-10-27
Engineer: Christ...
Provided to YouTube by Beggars Group Digital Ltd.
Convergence · Jonny Greenwood
Bodysong.
℗ 2003 XL Recordings Ltd
Released on: 2003-10-27
Engineer: Christian Wright
Engineer: Graeme Stewart
Producer: Graeme Stewart
Producer: Jonny Greenwood
Associated Performer: Jonny Greenwood
Engineer: Simon Gibson
Music Publisher: Warner Chappell Music Publishing Ltd.
Music Publisher: Harry Fox Agency
Music Publisher: CMRRA
Composer Lyricist: Jonny Greenwood
Auto-generated by YouTube.
https://wn.com/Convergence
Provided to YouTube by Beggars Group Digital Ltd.
Convergence · Jonny Greenwood
Bodysong.
℗ 2003 XL Recordings Ltd
Released on: 2003-10-27
Engineer: Christian Wright
Engineer: Graeme Stewart
Producer: Graeme Stewart
Producer: Jonny Greenwood
Associated Performer: Jonny Greenwood
Engineer: Simon Gibson
Music Publisher: Warner Chappell Music Publishing Ltd.
Music Publisher: Harry Fox Agency
Music Publisher: CMRRA
Composer Lyricist: Jonny Greenwood
Auto-generated by YouTube.
- published: 02 Jan 2020
- views: 66193
4:59
Convergent and divergent sequences | Series | AP Calculus BC | Khan Academy
Courses on Khan Academy are always 100% free. Start practicing—and saving your progress—now: https://www.khanacademy.org/math/ap-calculus-bc/bc-series-new/bc-1...
Courses on Khan Academy are always 100% free. Start practicing—and saving your progress—now: https://www.khanacademy.org/math/ap-calculus-bc/bc-series-new/bc-10-1/v/convergent-and-divergent-sequences
Using the recursive formula of a sequence to find its fifth term. Created by Sal Khan.
Practice this lesson yourself on KhanAcademy.org right now: https://www.khanacademy.org/math/ap-calculus-bc/bc-series/bc-inf-sequences/e/convergence-and-divergence-of-sequences?utm_source=YT&utm;_medium=Desc&utm;_campaign=APCalculusBC
Watch the next lesson: https://www.khanacademy.org/math/ap-calculus-bc/bc-series/bc-inf-sequences/v/identifying-sequence-convergence-divergence?utm_source=YT&utm;_medium=Desc&utm;_campaign=APCalculusBC
Missed the previous lesson? https://www.khanacademy.org/math/ap-calculus-bc/bc-series/bc-inf-sequences/v/term-of-recursive-sequence?utm_source=YT&utm;_medium=Desc&utm;_campaign=APCalculusBC
AP Calculus BC on Khan Academy: Learn AP Calculus BC - everything from AP Calculus AB plus a few extra goodies, such as Taylor series, to prepare you for the AP Test
About Khan Academy: Khan Academy is a nonprofit with a mission to provide a free, world-class education for anyone, anywhere. We believe learners of all ages should have unlimited access to free educational content they can master at their own pace. We use intelligent software, deep data analytics and intuitive user interfaces to help students and teachers around the world. Our resources cover preschool through early college education, including math, biology, chemistry, physics, economics, finance, history, grammar and more. We offer free personalized SAT test prep in partnership with the test developer, the College Board. Khan Academy has been translated into dozens of languages, and 100 million people use our platform worldwide every year. For more information, visit www.khanacademy.org, join us on Facebook or follow us on Twitter at @khanacademy. And remember, you can learn anything.
For free. For everyone. Forever. #YouCanLearnAnything
Subscribe to Khan Academy's AP Calculus BC channel: https://www.youtube.com/channel/UC5A2DBjjUVNz8axD-90jdfQ?sub_confirmation=1
Subscribe to Khan Academy: https://www.youtube.com/subscription_center?add_user=khanacademy
https://wn.com/Convergent_And_Divergent_Sequences_|_Series_|_Ap_Calculus_Bc_|_Khan_Academy
Courses on Khan Academy are always 100% free. Start practicing—and saving your progress—now: https://www.khanacademy.org/math/ap-calculus-bc/bc-series-new/bc-10-1/v/convergent-and-divergent-sequences
Using the recursive formula of a sequence to find its fifth term. Created by Sal Khan.
Practice this lesson yourself on KhanAcademy.org right now: https://www.khanacademy.org/math/ap-calculus-bc/bc-series/bc-inf-sequences/e/convergence-and-divergence-of-sequences?utm_source=YT&utm;_medium=Desc&utm;_campaign=APCalculusBC
Watch the next lesson: https://www.khanacademy.org/math/ap-calculus-bc/bc-series/bc-inf-sequences/v/identifying-sequence-convergence-divergence?utm_source=YT&utm;_medium=Desc&utm;_campaign=APCalculusBC
Missed the previous lesson? https://www.khanacademy.org/math/ap-calculus-bc/bc-series/bc-inf-sequences/v/term-of-recursive-sequence?utm_source=YT&utm;_medium=Desc&utm;_campaign=APCalculusBC
AP Calculus BC on Khan Academy: Learn AP Calculus BC - everything from AP Calculus AB plus a few extra goodies, such as Taylor series, to prepare you for the AP Test
About Khan Academy: Khan Academy is a nonprofit with a mission to provide a free, world-class education for anyone, anywhere. We believe learners of all ages should have unlimited access to free educational content they can master at their own pace. We use intelligent software, deep data analytics and intuitive user interfaces to help students and teachers around the world. Our resources cover preschool through early college education, including math, biology, chemistry, physics, economics, finance, history, grammar and more. We offer free personalized SAT test prep in partnership with the test developer, the College Board. Khan Academy has been translated into dozens of languages, and 100 million people use our platform worldwide every year. For more information, visit www.khanacademy.org, join us on Facebook or follow us on Twitter at @khanacademy. And remember, you can learn anything.
For free. For everyone. Forever. #YouCanLearnAnything
Subscribe to Khan Academy's AP Calculus BC channel: https://www.youtube.com/channel/UC5A2DBjjUVNz8axD-90jdfQ?sub_confirmation=1
Subscribe to Khan Academy: https://www.youtube.com/subscription_center?add_user=khanacademy
- published: 15 Feb 2013
- views: 905528
2:08
Convergence: Courage in a Crisis | Official Trailer | Netflix
An epic collaboration that spans eight countries and nine individual stories, Convergence reveals the power of compassion and community in the face of a crisis....
An epic collaboration that spans eight countries and nine individual stories, Convergence reveals the power of compassion and community in the face of a crisis. Only on Netflix October 12.
Featuring the original song "Beautiful Life" from Michael Kiwanuka.
SUBSCRIBE: http://bit.ly/29qBUt7
About Netflix:
Netflix is the world's leading streaming entertainment service with over 209 million paid memberships in over 190 countries enjoying TV series, documentaries and feature films across a wide variety of genres and languages. Members can watch as much as they want, anytime, anywhere, on any internet-connected screen. Members can play, pause and resume watching, all without commercials or commitments.
Convergence: Courage in a Crisis | Official Trailer | Netflix
https://youtube.com/Netflix
While COVID-19 exacerbates vulnerabilities across the world, unsung heroes in all levels of society help the tide turn toward a brighter future.
https://wn.com/Convergence_Courage_In_A_Crisis_|_Official_Trailer_|_Netflix
An epic collaboration that spans eight countries and nine individual stories, Convergence reveals the power of compassion and community in the face of a crisis. Only on Netflix October 12.
Featuring the original song "Beautiful Life" from Michael Kiwanuka.
SUBSCRIBE: http://bit.ly/29qBUt7
About Netflix:
Netflix is the world's leading streaming entertainment service with over 209 million paid memberships in over 190 countries enjoying TV series, documentaries and feature films across a wide variety of genres and languages. Members can watch as much as they want, anytime, anywhere, on any internet-connected screen. Members can play, pause and resume watching, all without commercials or commitments.
Convergence: Courage in a Crisis | Official Trailer | Netflix
https://youtube.com/Netflix
While COVID-19 exacerbates vulnerabilities across the world, unsung heroes in all levels of society help the tide turn toward a brighter future.
- published: 27 Sep 2021
- views: 102777
5:36
Convergence
Provided to YouTube by Base79
Convergence · Random Factor
Convergence
℗ 2020Vision
Released on: 2004-01-01
Auto-generated by YouTube.
Provided to YouTube by Base79
Convergence · Random Factor
Convergence
℗ 2020Vision
Released on: 2004-01-01
Auto-generated by YouTube.
https://wn.com/Convergence
Provided to YouTube by Base79
Convergence · Random Factor
Convergence
℗ 2020Vision
Released on: 2004-01-01
Auto-generated by YouTube.
- published: 10 Jun 2016
- views: 58707
28:52
Infinite Series(Part-I): Convergence in hindi
This video is useful for students of BSc/MSc Mathematics students. Also for students preparing IIT-JAM, GATE, CSIR-NET and other exams.
उम्मीद हे आपको ये videos...
This video is useful for students of BSc/MSc Mathematics students. Also for students preparing IIT-JAM, GATE, CSIR-NET and other exams.
उम्मीद हे आपको ये videos पसंद आ रही होगी, यदि कोई भी doubt या Problem हो तो शुरआत में आप callme4 app की ID "gr8bhagwan@cm4" पर फ़ोन कर सकते है. और पसंद वाली वीडियो को Like, Shear और सब्सक्राइब (Subscribe) करना न भूले, इससे क्रिएटर (Creator) को और ज़्यादा काम करने की प्रेरणा मिलती हे
keep support us to create "Skilled & educated India" we request you to visit & subscribe our Associated Channels to give us more strength.
#01 MotorLAB Ev's (For Electrical)
https://www.youtube.com/c/MotorLabEvs...
#02 Dailyinnovation4life (For Civil Engg.)
https://www.youtube.com/channel/UC75l...
#03 Vedam Compititive classes
https://www.youtube.com/channel/UCASK...
#04 Gr8Macrame Art (Home Science)
https://www.youtube.com/channel/UCOrT...
#05 JaipalVishwakarma (For School Maths)
https://www.youtube.com/channel/UCt6Q...
#06 Craft4model (For Automobile)
https://www.youtube.com/c/Craft4Model
..
मिलते हे अगली Video में.
धन्यवाद, जय हिन्द
https://wn.com/Infinite_Series(Part_I)_Convergence_In_Hindi
This video is useful for students of BSc/MSc Mathematics students. Also for students preparing IIT-JAM, GATE, CSIR-NET and other exams.
उम्मीद हे आपको ये videos पसंद आ रही होगी, यदि कोई भी doubt या Problem हो तो शुरआत में आप callme4 app की ID "gr8bhagwan@cm4" पर फ़ोन कर सकते है. और पसंद वाली वीडियो को Like, Shear और सब्सक्राइब (Subscribe) करना न भूले, इससे क्रिएटर (Creator) को और ज़्यादा काम करने की प्रेरणा मिलती हे
keep support us to create "Skilled & educated India" we request you to visit & subscribe our Associated Channels to give us more strength.
#01 MotorLAB Ev's (For Electrical)
https://www.youtube.com/c/MotorLabEvs...
#02 Dailyinnovation4life (For Civil Engg.)
https://www.youtube.com/channel/UC75l...
#03 Vedam Compititive classes
https://www.youtube.com/channel/UCASK...
#04 Gr8Macrame Art (Home Science)
https://www.youtube.com/channel/UCOrT...
#05 JaipalVishwakarma (For School Maths)
https://www.youtube.com/channel/UCt6Q...
#06 Craft4model (For Automobile)
https://www.youtube.com/c/Craft4Model
..
मिलते हे अगली Video में.
धन्यवाद, जय हिन्द
- published: 04 Sep 2016
- views: 828080
-
GSS Fall 2016 - Giovanni Gravina: An Introduction to Gamma-convergence
In this talk we will look at the so-called gamma-convergence, a notion of convergence for functionals which is particularly suited for problems in the calculus of variations. We'll start by motivating the definition and we'll prove properties of gamma-limits. Time permitting, we'll study the gamma-convergence of the Dirichlet energy on a perforated domain to investigate the behavior of solutions to Poisson's equation as the perforation vanishes.
published: 09 Nov 2016
-
Gamma Convergence (Lecture 2) by A K Nandakumaran
PROGRAM: MULTI-SCALE ANALYSIS AND THEORY OF HOMOGENIZATION
ORGANIZERS: Patrizia Donato, Editha Jose, Akambadath
Nandakumaran and Daniel Onofrei
DATE: 26 August 2019 to 06 September 2019
VENUE: Madhava Lecture Hall, ICTS, Bangalore
Homogenization is a mathematical procedure to understand the multi-scale analysis of various phenomena modeled by partial differential equations (PDEs). It is a relatively new area and has tremendous applications in various branches of engineering sciences like material science, porous media, the study of vibrations of thin structures, composite materials to name a few. Indeed, homogenization can be viewed as a process of understanding a heterogeneous media (where the heterogeneities are at the microscopic level like in composite materials) by a homogeneous ...
published: 31 Dec 2019
-
Gamma Convergence | Lec#2 on Calculus of Variations | Shah Faisal | www.mathsvolunteers.com
This lecture was delivered on September 24, 2022 to audience of Maths Volunteers.
The Lecture include:
1. Recap of lecture#1 (Classical approach- based on solving the Euler-Lagrange equation, and Direct Methods- based on extracting convergent sub-sequences out of a minimizing
2. Gamma convergence (definition, properties and theorems)
3. Comparison with other type of convergences
4. Finite-dimensional reduction
For more details and detailed version of the slides visit course page: https://www.mathsvolunteers.com/services/short-courses/calculus-of-variations
© All rights reserve by Maths Volunteers
Reference: Gianni Maso, An Introduction to Gamma-Convergence, Springer.
Abstract:
"In the previous lecture, I explained two techniques for studying the existence and computing minimize...
published: 28 Jul 2023
-
Gamma Function and Convergence ||Maths for Graduates
#RealAnalysishandwrittennotes
Whatsapp @9451434163 for more details.
or click below
Whatsapp
http://wa.me/+919451434163
Facebook
https://www.facebook.com/mathsforgraduates
Instagram
https://instagram.com/mathsforgraduates
Telegram
https://t.me/mathsforgraduates
For full Course click here:
https://www.youtube.com/playlist?list=PLbwJuBHc3YzUIgPk82CIm-doYjZa_SeKe
published: 19 Nov 2023
-
A Gamma-convergence result and an application to the Monge-Ampère gravitational model
Speaker: Luigi Ambrosio, Scuola Normale Superiore
2020 Fields Medal Symposium
http://www.fields.utoronto.ca/activities/20-21/fieldsmedalsym
Abstract: I illustrate a Gamma-convergence result for nonsmooth action functionals involving the minimal selection of the subdifferential of convex functions. The result has been motivated by the study of a discrete version of the MA gravitational model. Joint work with A.Baradat and Y.Brenier.
published: 20 Oct 2020
-
Matias Delgadino: Mean field limit by Gamma convergence
The lecture was held within the framework of the Hausdorff Trimester Program: Kinetic Theory
Abstract: In this work we give a proof of the mean-field limit for λ-convex potentials using a purely variational viewpoint. We take advantage that all evolutions of the involved quantities can be written as gradient flows of functionals at different levels: in the set of symmetric probability measures on N variables and in the set of probability measures on probability measures. This basic fact allows us to rely on Γ-convergence tools for gradient flows to finish the proof by identifying the limits of the different terms in the Evolutionary Variational Inequalities (EVIs) associated to each gradient flow. The λ-convexity of the potentials is crucial to identify uniquely the limits and in order to derive...
published: 28 May 2019
-
Davini, C — Composite Thin Walled Beams by Γ-Convergence
Composite Thin Walled Beams by Γ-Convergence
Talk by Cesare Davini from the University of Udine during the 52nd Meeting of the Society for Natural Philosophy (SNP), which was held in the Pedro Calmon Room at the Praia Vermelha Campus of the Federal University of Rio de Janeiro from October 22 to 24, 2014.
published: 30 Oct 2015
-
Convergence of Gamma Function
Proof of the Convergence of the Gamma Function for all real x greater than 0.
published: 05 Feb 2024
-
MAT 2420 Lecture 20 10 31 2024
published: 04 Nov 2024
-
Gamma Convergence (Lecture 3) by Nandakumar
PROGRAM: MULTI-SCALE ANALYSIS AND THEORY OF HOMOGENIZATION
ORGANIZERS: Patrizia Donato, Editha Jose, Akambadath
Nandakumaran and Daniel Onofrei
DATE: 26 August 2019 to 06 September 2019
VENUE: Madhava Lecture Hall, ICTS, Bangalore
Homogenization is a mathematical procedure to understand the multi-scale analysis of various phenomena modeled by partial differential equations (PDEs). It is a relatively new area and has tremendous applications in various branches of engineering sciences like material science, porous media, the study of vibrations of thin structures, composite materials to name a few. Indeed, homogenization can be viewed as a process of understanding a heterogeneous media (where the heterogeneities are at the microscopic level like in composite materials) by a homogeneous ...
published: 31 Dec 2019
1:13:34
GSS Fall 2016 - Giovanni Gravina: An Introduction to Gamma-convergence
In this talk we will look at the so-called gamma-convergence, a notion of convergence for functionals which is particularly suited for problems in the calculus ...
In this talk we will look at the so-called gamma-convergence, a notion of convergence for functionals which is particularly suited for problems in the calculus of variations. We'll start by motivating the definition and we'll prove properties of gamma-limits. Time permitting, we'll study the gamma-convergence of the Dirichlet energy on a perforated domain to investigate the behavior of solutions to Poisson's equation as the perforation vanishes.
https://wn.com/Gss_Fall_2016_Giovanni_Gravina_An_Introduction_To_Gamma_Convergence
In this talk we will look at the so-called gamma-convergence, a notion of convergence for functionals which is particularly suited for problems in the calculus of variations. We'll start by motivating the definition and we'll prove properties of gamma-limits. Time permitting, we'll study the gamma-convergence of the Dirichlet energy on a perforated domain to investigate the behavior of solutions to Poisson's equation as the perforation vanishes.
- published: 09 Nov 2016
- views: 1265
1:30:07
Gamma Convergence (Lecture 2) by A K Nandakumaran
PROGRAM: MULTI-SCALE ANALYSIS AND THEORY OF HOMOGENIZATION
ORGANIZERS: Patrizia Donato, Editha Jose, Akambadath
Nandakumaran and Daniel Onofrei
DATE: 26 Augu...
PROGRAM: MULTI-SCALE ANALYSIS AND THEORY OF HOMOGENIZATION
ORGANIZERS: Patrizia Donato, Editha Jose, Akambadath
Nandakumaran and Daniel Onofrei
DATE: 26 August 2019 to 06 September 2019
VENUE: Madhava Lecture Hall, ICTS, Bangalore
Homogenization is a mathematical procedure to understand the multi-scale analysis of various phenomena modeled by partial differential equations (PDEs). It is a relatively new area and has tremendous applications in various branches of engineering sciences like material science, porous media, the study of vibrations of thin structures, composite materials to name a few. Indeed, homogenization can be viewed as a process of understanding a heterogeneous media (where the heterogeneities are at the microscopic level like in composite materials) by a homogeneous media. Mathematically, homogenization deals with the study of asymptotic analysis of the solutions of PDEs by obtaining the equation satisfied by the limit. This limit equation will characterize the bulk or overall behavior of the material, which does not consist of microscopic heterogeneities and can be solved or computed.
Topics to be covered in the workshop include the following but not limited to:
1. Multi-scale problems in applications
2. Introduction to homogenization
3. Techniques in homogenization
4. Recent trends
Plan and Schedule of the Program:
In this discussion meeting, we go through several examples to understand the homogenization procedure in a general perspective together with applications. We also present various mathematical techniques available and some details about the techniques. A tutorial cum problem-solving session will also be conducted so that beginners can learn the material rigorously. In this way, we can train local and international junior students and researchers by equipping them with the theory and applications of multi-scale analysis and homogenization. Furthermore, the speakers will also discuss some ongoing current research and new problems which can foster mentoring or collaboration between students and experts on the area of analysis of multi-scale phenomena.
In addition to the basic material of homogenization, the speakers will be presenting the recent results in their area of expertise and this will be an opportunity for the youngsters to get into this beautiful area of research. Further, every day, we are planning to have a tutorial cum problem-solving session through which the beginners can learn the material in a better way. To make the tutorials/training sessions more effective, we may form small groups and each group may be asked to do some specific material that they can present on the last day. Each small group may be trained by one of the speakers who are available for the entire workshop,
The schedule mainly consists of 4, one-hour lectures per day and a Tutorial cum Problem-Solving Session of one and a half hours which can be extended according to the requirement of the participants.
CONTACT US: math2019@icts.res.in
PROGRAM LINK: https://www.icts.res.in/program/math2019
Table of Contents (powered by https://videoken.com)
0:00:00 Gamma Convergence (Lecture 2)
0:03:50 Minimization problem
0:06:14 Alpha = inf F(y) [Minimal value; Minimal point; Minimal sequence]
0:09:16 Graphs
0:17:43 Coercine
0:22:45 Definition
0:25:53 Theorem: Assume F. X-is Coercine and lsc
0:29:38 Proof:
0:32:55 F is given by an integral functions
0:40:26 Problem Solution
0:46:54 Concept of derivative
0:57:04 Exercise: assume u E C2 (Omega)
1:01:56 Integral functions
1:04:34 Proposition: F is lsc in strong topology
1:10:27 Two other functions
1:13:33 Weak lower semi continuity
1:17:29 Homogenization
1:27:51 Next
https://wn.com/Gamma_Convergence_(Lecture_2)_By_A_K_Nandakumaran
PROGRAM: MULTI-SCALE ANALYSIS AND THEORY OF HOMOGENIZATION
ORGANIZERS: Patrizia Donato, Editha Jose, Akambadath
Nandakumaran and Daniel Onofrei
DATE: 26 August 2019 to 06 September 2019
VENUE: Madhava Lecture Hall, ICTS, Bangalore
Homogenization is a mathematical procedure to understand the multi-scale analysis of various phenomena modeled by partial differential equations (PDEs). It is a relatively new area and has tremendous applications in various branches of engineering sciences like material science, porous media, the study of vibrations of thin structures, composite materials to name a few. Indeed, homogenization can be viewed as a process of understanding a heterogeneous media (where the heterogeneities are at the microscopic level like in composite materials) by a homogeneous media. Mathematically, homogenization deals with the study of asymptotic analysis of the solutions of PDEs by obtaining the equation satisfied by the limit. This limit equation will characterize the bulk or overall behavior of the material, which does not consist of microscopic heterogeneities and can be solved or computed.
Topics to be covered in the workshop include the following but not limited to:
1. Multi-scale problems in applications
2. Introduction to homogenization
3. Techniques in homogenization
4. Recent trends
Plan and Schedule of the Program:
In this discussion meeting, we go through several examples to understand the homogenization procedure in a general perspective together with applications. We also present various mathematical techniques available and some details about the techniques. A tutorial cum problem-solving session will also be conducted so that beginners can learn the material rigorously. In this way, we can train local and international junior students and researchers by equipping them with the theory and applications of multi-scale analysis and homogenization. Furthermore, the speakers will also discuss some ongoing current research and new problems which can foster mentoring or collaboration between students and experts on the area of analysis of multi-scale phenomena.
In addition to the basic material of homogenization, the speakers will be presenting the recent results in their area of expertise and this will be an opportunity for the youngsters to get into this beautiful area of research. Further, every day, we are planning to have a tutorial cum problem-solving session through which the beginners can learn the material in a better way. To make the tutorials/training sessions more effective, we may form small groups and each group may be asked to do some specific material that they can present on the last day. Each small group may be trained by one of the speakers who are available for the entire workshop,
The schedule mainly consists of 4, one-hour lectures per day and a Tutorial cum Problem-Solving Session of one and a half hours which can be extended according to the requirement of the participants.
CONTACT US: math2019@icts.res.in
PROGRAM LINK: https://www.icts.res.in/program/math2019
Table of Contents (powered by https://videoken.com)
0:00:00 Gamma Convergence (Lecture 2)
0:03:50 Minimization problem
0:06:14 Alpha = inf F(y) [Minimal value; Minimal point; Minimal sequence]
0:09:16 Graphs
0:17:43 Coercine
0:22:45 Definition
0:25:53 Theorem: Assume F. X-is Coercine and lsc
0:29:38 Proof:
0:32:55 F is given by an integral functions
0:40:26 Problem Solution
0:46:54 Concept of derivative
0:57:04 Exercise: assume u E C2 (Omega)
1:01:56 Integral functions
1:04:34 Proposition: F is lsc in strong topology
1:10:27 Two other functions
1:13:33 Weak lower semi continuity
1:17:29 Homogenization
1:27:51 Next
- published: 31 Dec 2019
- views: 808
46:37
Gamma Convergence | Lec#2 on Calculus of Variations | Shah Faisal | www.mathsvolunteers.com
This lecture was delivered on September 24, 2022 to audience of Maths Volunteers.
The Lecture include:
1. Recap of lecture#1 (Classical approach- based on solv...
This lecture was delivered on September 24, 2022 to audience of Maths Volunteers.
The Lecture include:
1. Recap of lecture#1 (Classical approach- based on solving the Euler-Lagrange equation, and Direct Methods- based on extracting convergent sub-sequences out of a minimizing
2. Gamma convergence (definition, properties and theorems)
3. Comparison with other type of convergences
4. Finite-dimensional reduction
For more details and detailed version of the slides visit course page: https://www.mathsvolunteers.com/services/short-courses/calculus-of-variations
© All rights reserve by Maths Volunteers
Reference: Gianni Maso, An Introduction to Gamma-Convergence, Springer.
Abstract:
"In the previous lecture, I explained two techniques for studying the existence and computing minimizers of functionals: a classical method based on solving the Euler–Lagrange equation coming from the first variation of the functional, and a modern approach based on extracting convergent sub-sequences from minimizing sequences of the functional under consideration.
In this lecture, I will explain another direct technique in which instead of looking at the original functional, one can look at a nice perturbation of the original functional and extract information about the minimizers of the original functional by studying the perturbed one. Such a perturbation is known as a Gamma-perturbation. I will explain the notion of Gamma-perturbation with examples and demonstrate how one can recover some minimizers of a given functional via gamma-perturbations of the given functional."
#CalculusOfVariations
#EulerLagrangeEquation
#DirectMethods
#GammaConvergence
#FiniteDimensionalReduction
#MathsVolunteers
#ShortCourses
#LectureNotes
#GianniMaso
#GammaPerturbation
#Functionals
#Minimizers
#ConvergenceTheory
#MathematicsEducation
https://wn.com/Gamma_Convergence_|_Lec_2_On_Calculus_Of_Variations_|_Shah_Faisal_|_Www.Mathsvolunteers.Com
This lecture was delivered on September 24, 2022 to audience of Maths Volunteers.
The Lecture include:
1. Recap of lecture#1 (Classical approach- based on solving the Euler-Lagrange equation, and Direct Methods- based on extracting convergent sub-sequences out of a minimizing
2. Gamma convergence (definition, properties and theorems)
3. Comparison with other type of convergences
4. Finite-dimensional reduction
For more details and detailed version of the slides visit course page: https://www.mathsvolunteers.com/services/short-courses/calculus-of-variations
© All rights reserve by Maths Volunteers
Reference: Gianni Maso, An Introduction to Gamma-Convergence, Springer.
Abstract:
"In the previous lecture, I explained two techniques for studying the existence and computing minimizers of functionals: a classical method based on solving the Euler–Lagrange equation coming from the first variation of the functional, and a modern approach based on extracting convergent sub-sequences from minimizing sequences of the functional under consideration.
In this lecture, I will explain another direct technique in which instead of looking at the original functional, one can look at a nice perturbation of the original functional and extract information about the minimizers of the original functional by studying the perturbed one. Such a perturbation is known as a Gamma-perturbation. I will explain the notion of Gamma-perturbation with examples and demonstrate how one can recover some minimizers of a given functional via gamma-perturbations of the given functional."
#CalculusOfVariations
#EulerLagrangeEquation
#DirectMethods
#GammaConvergence
#FiniteDimensionalReduction
#MathsVolunteers
#ShortCourses
#LectureNotes
#GianniMaso
#GammaPerturbation
#Functionals
#Minimizers
#ConvergenceTheory
#MathematicsEducation
- published: 28 Jul 2023
- views: 202
13:20
Gamma Function and Convergence ||Maths for Graduates
#RealAnalysishandwrittennotes
Whatsapp @9451434163 for more details.
or click below
Whatsapp
http://wa.me/+919451434163
Facebook
https://www.facebook.com/math...
#RealAnalysishandwrittennotes
Whatsapp @9451434163 for more details.
or click below
Whatsapp
http://wa.me/+919451434163
Facebook
https://www.facebook.com/mathsforgraduates
Instagram
https://instagram.com/mathsforgraduates
Telegram
https://t.me/mathsforgraduates
For full Course click here:
https://www.youtube.com/playlist?list=PLbwJuBHc3YzUIgPk82CIm-doYjZa_SeKe
https://wn.com/Gamma_Function_And_Convergence_||Maths_For_Graduates
#RealAnalysishandwrittennotes
Whatsapp @9451434163 for more details.
or click below
Whatsapp
http://wa.me/+919451434163
Facebook
https://www.facebook.com/mathsforgraduates
Instagram
https://instagram.com/mathsforgraduates
Telegram
https://t.me/mathsforgraduates
For full Course click here:
https://www.youtube.com/playlist?list=PLbwJuBHc3YzUIgPk82CIm-doYjZa_SeKe
- published: 19 Nov 2023
- views: 325
48:22
A Gamma-convergence result and an application to the Monge-Ampère gravitational model
Speaker: Luigi Ambrosio, Scuola Normale Superiore
2020 Fields Medal Symposium
http://www.fields.utoronto.ca/activities/20-21/fieldsmedalsym
Abstract: I illust...
Speaker: Luigi Ambrosio, Scuola Normale Superiore
2020 Fields Medal Symposium
http://www.fields.utoronto.ca/activities/20-21/fieldsmedalsym
Abstract: I illustrate a Gamma-convergence result for nonsmooth action functionals involving the minimal selection of the subdifferential of convex functions. The result has been motivated by the study of a discrete version of the MA gravitational model. Joint work with A.Baradat and Y.Brenier.
https://wn.com/A_Gamma_Convergence_Result_And_An_Application_To_The_Monge_Ampère_Gravitational_Model
Speaker: Luigi Ambrosio, Scuola Normale Superiore
2020 Fields Medal Symposium
http://www.fields.utoronto.ca/activities/20-21/fieldsmedalsym
Abstract: I illustrate a Gamma-convergence result for nonsmooth action functionals involving the minimal selection of the subdifferential of convex functions. The result has been motivated by the study of a discrete version of the MA gravitational model. Joint work with A.Baradat and Y.Brenier.
- published: 20 Oct 2020
- views: 570
29:49
Matias Delgadino: Mean field limit by Gamma convergence
The lecture was held within the framework of the Hausdorff Trimester Program: Kinetic Theory
Abstract: In this work we give a proof of the mean-field limit for ...
The lecture was held within the framework of the Hausdorff Trimester Program: Kinetic Theory
Abstract: In this work we give a proof of the mean-field limit for λ-convex potentials using a purely variational viewpoint. We take advantage that all evolutions of the involved quantities can be written as gradient flows of functionals at different levels: in the set of symmetric probability measures on N variables and in the set of probability measures on probability measures. This basic fact allows us to rely on Γ-convergence tools for gradient flows to finish the proof by identifying the limits of the different terms in the Evolutionary Variational Inequalities (EVIs) associated to each gradient flow. The λ-convexity of the potentials is crucial to identify uniquely the limits and in order to derive the EVIs at each description level of the interacting particle system. This is joint work with J.A. Carrillo and G. Pavliotis.
https://wn.com/Matias_Delgadino_Mean_field_Limit_By_Gamma_Convergence
The lecture was held within the framework of the Hausdorff Trimester Program: Kinetic Theory
Abstract: In this work we give a proof of the mean-field limit for λ-convex potentials using a purely variational viewpoint. We take advantage that all evolutions of the involved quantities can be written as gradient flows of functionals at different levels: in the set of symmetric probability measures on N variables and in the set of probability measures on probability measures. This basic fact allows us to rely on Γ-convergence tools for gradient flows to finish the proof by identifying the limits of the different terms in the Evolutionary Variational Inequalities (EVIs) associated to each gradient flow. The λ-convexity of the potentials is crucial to identify uniquely the limits and in order to derive the EVIs at each description level of the interacting particle system. This is joint work with J.A. Carrillo and G. Pavliotis.
- published: 28 May 2019
- views: 386
54:16
Davini, C — Composite Thin Walled Beams by Γ-Convergence
Composite Thin Walled Beams by Γ-Convergence
Talk by Cesare Davini from the University of Udine during the 52nd Meeting of the Society for Natural Philosophy (...
Composite Thin Walled Beams by Γ-Convergence
Talk by Cesare Davini from the University of Udine during the 52nd Meeting of the Society for Natural Philosophy (SNP), which was held in the Pedro Calmon Room at the Praia Vermelha Campus of the Federal University of Rio de Janeiro from October 22 to 24, 2014.
https://wn.com/Davini,_C_—_Composite_Thin_Walled_Beams_By_Γ_Convergence
Composite Thin Walled Beams by Γ-Convergence
Talk by Cesare Davini from the University of Udine during the 52nd Meeting of the Society for Natural Philosophy (SNP), which was held in the Pedro Calmon Room at the Praia Vermelha Campus of the Federal University of Rio de Janeiro from October 22 to 24, 2014.
- published: 30 Oct 2015
- views: 173
14:30
Convergence of Gamma Function
Proof of the Convergence of the Gamma Function for all real x greater than 0.
Proof of the Convergence of the Gamma Function for all real x greater than 0.
https://wn.com/Convergence_Of_Gamma_Function
Proof of the Convergence of the Gamma Function for all real x greater than 0.
- published: 05 Feb 2024
- views: 722
1:12:32
Gamma Convergence (Lecture 3) by Nandakumar
PROGRAM: MULTI-SCALE ANALYSIS AND THEORY OF HOMOGENIZATION
ORGANIZERS: Patrizia Donato, Editha Jose, Akambadath
Nandakumaran and Daniel Onofrei
DATE: 26 Augu...
PROGRAM: MULTI-SCALE ANALYSIS AND THEORY OF HOMOGENIZATION
ORGANIZERS: Patrizia Donato, Editha Jose, Akambadath
Nandakumaran and Daniel Onofrei
DATE: 26 August 2019 to 06 September 2019
VENUE: Madhava Lecture Hall, ICTS, Bangalore
Homogenization is a mathematical procedure to understand the multi-scale analysis of various phenomena modeled by partial differential equations (PDEs). It is a relatively new area and has tremendous applications in various branches of engineering sciences like material science, porous media, the study of vibrations of thin structures, composite materials to name a few. Indeed, homogenization can be viewed as a process of understanding a heterogeneous media (where the heterogeneities are at the microscopic level like in composite materials) by a homogeneous media. Mathematically, homogenization deals with the study of asymptotic analysis of the solutions of PDEs by obtaining the equation satisfied by the limit. This limit equation will characterize the bulk or overall behavior of the material, which does not consist of microscopic heterogeneities and can be solved or computed.
Topics to be covered in the workshop include the following but not limited to:
1. Multi-scale problems in applications
2. Introduction to homogenization
3. Techniques in homogenization
4. Recent trends
Plan and Schedule of the Program:
In this discussion meeting, we go through several examples to understand the homogenization procedure in a general perspective together with applications. We also present various mathematical techniques available and some details about the techniques. A tutorial cum problem-solving session will also be conducted so that beginners can learn the material rigorously. In this way, we can train local and international junior students and researchers by equipping them with the theory and applications of multi-scale analysis and homogenization. Furthermore, the speakers will also discuss some ongoing current research and new problems which can foster mentoring or collaboration between students and experts on the area of analysis of multi-scale phenomena.
In addition to the basic material of homogenization, the speakers will be presenting the recent results in their area of expertise and this will be an opportunity for the youngsters to get into this beautiful area of research. Further, every day, we are planning to have a tutorial cum problem-solving session through which the beginners can learn the material in a better way. To make the tutorials/training sessions more effective, we may form small groups and each group may be asked to do some specific material that they can present on the last day. Each small group may be trained by one of the speakers who are available for the entire workshop,
The schedule mainly consists of 4, one-hour lectures per day and a Tutorial cum Problem-Solving Session of one and a half hours which can be extended according to the requirement of the participants.
CONTACT US: math2019@icts.res.in
PROGRAM LINK: https://www.icts.res.in/program/math2019
Table of Contents (powered by https://videoken.com)
0:00:00 Gamma Convergence (Lecture 3)
0:03:09 Examples
0:09:43 Correct Function
0:11:40 Sketch the graph
0:18:16 Relaxation
0:22:40 Proposition: Arbitrary Sup (lower semi continuous) is lower semi continuous
0:30:05 Theorem:
0:36:38 Comparison with Continuity F
0:39:29 Theorem: Let F . X -R be Covicine
0:47:47 Assume f
0:50:19 First polar function
0:55:47 Def (Gamma - Convergence)
0:57:44 Comparison with point wise convergence
1:02:59 Exercise
https://wn.com/Gamma_Convergence_(Lecture_3)_By_Nandakumar
PROGRAM: MULTI-SCALE ANALYSIS AND THEORY OF HOMOGENIZATION
ORGANIZERS: Patrizia Donato, Editha Jose, Akambadath
Nandakumaran and Daniel Onofrei
DATE: 26 August 2019 to 06 September 2019
VENUE: Madhava Lecture Hall, ICTS, Bangalore
Homogenization is a mathematical procedure to understand the multi-scale analysis of various phenomena modeled by partial differential equations (PDEs). It is a relatively new area and has tremendous applications in various branches of engineering sciences like material science, porous media, the study of vibrations of thin structures, composite materials to name a few. Indeed, homogenization can be viewed as a process of understanding a heterogeneous media (where the heterogeneities are at the microscopic level like in composite materials) by a homogeneous media. Mathematically, homogenization deals with the study of asymptotic analysis of the solutions of PDEs by obtaining the equation satisfied by the limit. This limit equation will characterize the bulk or overall behavior of the material, which does not consist of microscopic heterogeneities and can be solved or computed.
Topics to be covered in the workshop include the following but not limited to:
1. Multi-scale problems in applications
2. Introduction to homogenization
3. Techniques in homogenization
4. Recent trends
Plan and Schedule of the Program:
In this discussion meeting, we go through several examples to understand the homogenization procedure in a general perspective together with applications. We also present various mathematical techniques available and some details about the techniques. A tutorial cum problem-solving session will also be conducted so that beginners can learn the material rigorously. In this way, we can train local and international junior students and researchers by equipping them with the theory and applications of multi-scale analysis and homogenization. Furthermore, the speakers will also discuss some ongoing current research and new problems which can foster mentoring or collaboration between students and experts on the area of analysis of multi-scale phenomena.
In addition to the basic material of homogenization, the speakers will be presenting the recent results in their area of expertise and this will be an opportunity for the youngsters to get into this beautiful area of research. Further, every day, we are planning to have a tutorial cum problem-solving session through which the beginners can learn the material in a better way. To make the tutorials/training sessions more effective, we may form small groups and each group may be asked to do some specific material that they can present on the last day. Each small group may be trained by one of the speakers who are available for the entire workshop,
The schedule mainly consists of 4, one-hour lectures per day and a Tutorial cum Problem-Solving Session of one and a half hours which can be extended according to the requirement of the participants.
CONTACT US: math2019@icts.res.in
PROGRAM LINK: https://www.icts.res.in/program/math2019
Table of Contents (powered by https://videoken.com)
0:00:00 Gamma Convergence (Lecture 3)
0:03:09 Examples
0:09:43 Correct Function
0:11:40 Sketch the graph
0:18:16 Relaxation
0:22:40 Proposition: Arbitrary Sup (lower semi continuous) is lower semi continuous
0:30:05 Theorem:
0:36:38 Comparison with Continuity F
0:39:29 Theorem: Let F . X -R be Covicine
0:47:47 Assume f
0:50:19 First polar function
0:55:47 Def (Gamma - Convergence)
0:57:44 Comparison with point wise convergence
1:02:59 Exercise
- published: 31 Dec 2019
- views: 424
 be a topological space and
be a topological space and  a sequence of functionals on
a sequence of functionals on  . Then
. Then  are said to
are said to  -converge to the
-converge to the  -limit
-limit  if the following two conditions hold:
if the following two conditions hold: such that
such that  as
as  ,
, , there is a sequence
, there is a sequence  converging to
converging to  such that
such that provides an asymptotic common lower bound for the
provides an asymptotic common lower bound for the  . The second condition means that this lower bound is optimal.
. The second condition means that this lower bound is optimal.
 -converge to
-converge to  , and
, and  is a minimizer for
is a minimizer for  , then every cluster point of the sequence
, then every cluster point of the sequence  is a minimizer of
is a minimizer of  .
. -limits are always lower semicontinuous.
-limits are always lower semicontinuous. -convergence is stable under continuous perturbations: If
-convergence is stable under continuous perturbations: If 
 -converges to
-converges to  and
and 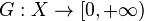 is continuous, then
is continuous, then  will
will  -converge to
-converge to  .
. does not necessarily
does not necessarily  -converge to
-converge to  , but to the relaxation of
, but to the relaxation of  , the largest lower semicontinuous functional below
, the largest lower semicontinuous functional below  .
.
























































