- published: 24 Feb 2013
- views: 17566
-
remove the playlistNonlinear
- remove the playlistNonlinear
- published: 20 Sep 2014
- views: 11939
- published: 09 Sep 2012
- views: 62509
- published: 31 Jan 2014
- views: 55489
- published: 22 Aug 2015
- views: 3123
- published: 05 Apr 2015
- views: 1558
- published: 04 Jun 2013
- views: 66102
- published: 16 Mar 2016
- views: 756
- published: 26 Oct 2010
- views: 9749
- published: 03 Sep 2015
- views: 2415
In mathematics, a nonlinear system is one that does not satisfy the superposition principle, or one whose output is not directly proportional to its input; a linear system fulfills these conditions. In other words, a nonlinear system is any problem where the variable(s) to be solved for cannot be written as a linear combination of independent components. A nonhomogeneous system, which is linear apart from the presence of a function of the independent variables, is nonlinear according to a strict definition, but such systems are usually studied alongside linear systems, because they can be transformed to a linear system of multiple variables.
Nonlinear problems are of interest to engineers, physicists and mathematicians because most physical systems are inherently nonlinear in nature. Nonlinear equations are difficult to solve and give rise to interesting phenomena such as chaos. The weather is famously chaotic, where simple changes in one part of the system produce complex effects throughout.
This article is licensed under the Creative Commons Attribution-ShareAlike 3.0 Unported License, which means that you can copy and modify it as long as the entire work (including additions) remains under this license.
- Loading...

-
 1:57
1:57How to Distinguish Between Linear & Nonlinear : Math Teacher Tips
How to Distinguish Between Linear & Nonlinear : Math Teacher TipsHow to Distinguish Between Linear & Nonlinear : Math Teacher Tips
Subscribe Now: http://www.youtube.com/subscription_center?add_user=ehoweducation Watch More: http://www.youtube.com/ehoweducation Distinguishing between the terms linear and non-linear is pretty straightforward if you just keep a few important things in mind. Distinguish between linear and non-linear with help from a mathematics educator with over a decade of experience in this free video clip. Expert: Jimmy Chang Filmmaker: Christopher Rokosz Series Description: Teaching math always requires that you take the comfort level and learning abilities of your students in mind when planning lessons. Get tips specifically geared towards teaching mathematics with help from a mathematics educator with over a decade of experience in this free video series. -
 5:49
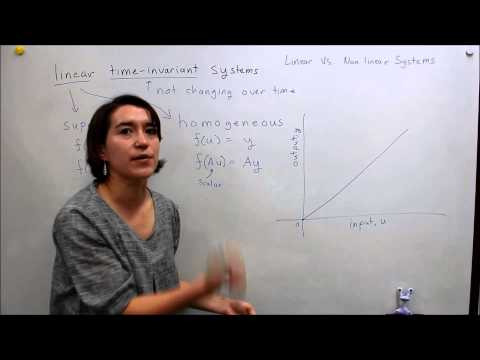
5:49Intro to Control - 4.3 Linear Versus Nonlinear Systems
Intro to Control - 4.3 Linear Versus Nonlinear SystemsIntro to Control - 4.3 Linear Versus Nonlinear Systems
Defining a linear system. Talking about the difference between linear and nonlinear systems. -
 7:05
7:05Linear or Non-Linear Functions
Linear or Non-Linear Functions -
 3:26
3:26ODE | Linear versus nonlinear
ODE | Linear versus nonlinearODE | Linear versus nonlinear
Examples and explanations for a course in ordinary differential equations. ODE playlist: http://www.youtube.com/playlist?list=PLwIFHT1FWIUJYuP5y6YEM4WWrY4kEmIuS In this video we explain what a linear differential equation looks like, and give some examples of nonlinear differential equations. This is done by analogy with linear and nonlinear algebraic equations. We give the general form for a first order and a second order linear differential equation. -
 15:05
15:05Nonlinear Model Fitting using Excel
Nonlinear Model Fitting using ExcelNonlinear Model Fitting using Excel
Using Excel's Solver tool to estimate non-linear model fitting parameters. -
 9:14
9:14Nonlinear Regression in Microsoft Excel
Nonlinear Regression in Microsoft ExcelNonlinear Regression in Microsoft Excel
A three parameter (a,b,c) model y = a + b/x + c ln(x) is fit to a set of data with the Excel solver add-in. This tutorial walks through the process of installing the solver, setting up the objective (normalized sum of squared errors), and adjusting the parameter values to minimize the SSE. -
 6:47
6:47Nonlinear Systems: 3 Nonlinearity Overview
Nonlinear Systems: 3 Nonlinearity OverviewNonlinear Systems: 3 Nonlinearity Overview
Having now laid down our foundations this is where our discussion on nonlinearity really starts. We will talk about why and how linear systems theory breaks down as soon as we have some set of relations within a system that are non-additive, which appears to be often the case in the real world, we also look at how feedback loops over time work to defy the homogeneity principle with the net result being nonlinear behavior. For full courses, transcriptions & downloads please see: http://complexitylearning.io Twitter: https://twitter.com/complexitylearn Facebook: https://goo.gl/ggxGMT G+: https://goo.gl/G6zTMg Transcription excerpt: Although it is often said that nonlinear systems describe the vast majority of phenomena in our world, they have unfortunately been designated as alternatives, being defined by what they are not, it might be of value to start our discussion by asking why is this so? The real world we live in is inherently complex and nonlinear, but from a scientific perspective all we have is our models to try and understand it, these models have inevitably started simple and developed to become more complex and sophisticated representations. When we say simple in this case, we mean things that are the product of direct cause and effect interactions, with these simple interactions we can draw a direct line between cause and effect and thus defined a linear relation. For centuries science and mathematics has been focused upon these simple linear interactions and orderly geometric forms, that can be describe in beautifully compact equations, not so much because this is how the world is, but more because they are by far the easiest phenomena for us to encode in our language of mathematics and science. It is only in the past few decades that scientist have begun to approach the world of systems that are not linear, thus their late arrival on the scene and our lack of understanding of what they really are has lumped them with being defined by what they are not. In the previous section we discussed the key characteristics of linear systems, what is called the superposition principals, we can then defined nonlinear systems as those the defy the superposition principals, meaning with nonlinear phenomena the principals of homogeneity and additively break down, but lets take a closer look at why this is so. Starting with additively, as we have already discussed additively states that when we put two or more components together, the resulting combined system will be nothing more than a simple addition of each component’s properties in isolation. The additively principal, as attractively simple as it is, breaks down in nonlinear systems, because the way we put things together and the type of things we put together effect the interactions that make the overall product of the components combination more or less than a simple additive function and thus defies our additively principal and we call it nonlinear. There are many examples of this such as putting two creatures together, depending which type of creatures we choose, we will get qualitatively different types of interaction between them, that may well make the combination non- additive, Bees and flowers create synergistic interactions or lions and deer interacting through relations of predator and prey, both of these represent either super or sub-linear interactions. -
 2:26
2:26Linear and nonlinear functions (example 1) | 8th grade | Khan Academy
Linear and nonlinear functions (example 1) | 8th grade | Khan AcademyLinear and nonlinear functions (example 1) | 8th grade | Khan Academy
Practice this lesson yourself on KhanAcademy.org right now: https://www.khanacademy.org/math/cc-eighth-grade-math/cc-8th-linear-equations-functions/linear-nonlinear-functions-tut/e/linear-non-linear-functions?utm_source=YT&utm;_medium=Desc&utm;_campaign=8thgrade Watch the next lesson: https://www.khanacademy.org/math/cc-eighth-grade-math/cc-8th-linear-equations-functions/linear-nonlinear-functions-tut/v/linear-and-nonlinear-functions-example-1?utm_source=YT&utm;_medium=Desc&utm;_campaign=8thgrade Missed the previous lesson? https://www.khanacademy.org/math/cc-eighth-grade-math/cc-8th-linear-equations-functions/linear-nonlinear-functions-tut/v/recognizing-linear-functions?utm_source=YT&utm;_medium=Desc&utm;_campaign=8thgrade 8th grade on Khan Academy: 8th grade is all about tackling the meat of algebra and getting exposure to some of the foundational concepts in geometry. If you get this stuff (and you should because you're incredibly persistent), the rest of your life will be easy. Okay, maybe not your whole life, but at least your mathematical life. Seriously, if you really get the equations and functions stuff we cover here, most of high school will feel intuitive, even relaxing. (Content was selected for this grade level based on a typical curriculum in the United States.) About Khan Academy: Khan Academy offers practice exercises, instructional videos, and a personalized learning dashboard that empower learners to study at their own pace in and outside of the classroom. We tackle math, science, computer programming, history, art history, economics, and more. Our math missions guide learners from kindergarten to calculus using state-of-the-art, adaptive technology that identifies strengths and learning gaps. We've also partnered with institutions like NASA, The Museum of Modern Art, The California Academy of Sciences, and MIT to offer specialized content. For free. For everyone. Forever. #YouCanLearnAnything Subscribe to Khan Academy’s 8th grade channel: https://www.youtube.com/channel/UCq341CBxj87C7AY_zu2qPlQ?sub_confirmation=1 Subscribe to Khan Academy: https://www.youtube.com/subscription_center?add_user=khanacademy -
 4:39
4:39Little Tybee's Josh Martin presents: Try This! Nonlinear Pulloffs
Little Tybee's Josh Martin presents: Try This! Nonlinear PulloffsLittle Tybee's Josh Martin presents: Try This! Nonlinear Pulloffs
Here's Little Tybee's Josh Martin with a new lesson video for some non-linear pulloff techniques that he likes to employ in his playing. Little Tybee is currently running a Kickstarter campaign for their new record! Check out www.littletybee.com to find out more! -
 4:15
4:15Nonlinear - Stacce
Nonlinear - StacceNonlinear - Stacce
Official video. Song "Stacce", extracted from the album "Sei Mejo Te", out in 2010 on Subsound Records. Video produced by Tekla, directed by Gianluca Orrù. Assistant: Veronica Pianetti HDSL Operator: Fabrizio Cecioni www.teklastudio.com ----------------------------------------------------------------------------- "..così può capitare che 'Stacce' inizi rap, prosegua con un bridge più emo dei 30 Seconds to Mars e finisca con una cavalcata hardcore". Rockerilla -
 7:09
7:09Solve Nonlinear Equations with MATLAB
Solve Nonlinear Equations with MATLABSolve Nonlinear Equations with MATLAB
MATLAB solves nonlinear equations either symbolically or numerically with solvers such as "fsolve". This example demonstrates how to obtain a solution to sets of nonlinear equations. -
 14:52
14:52Statistics - Multiple & Nonlinear Regression
Statistics - Multiple & Nonlinear RegressionStatistics - Multiple & Nonlinear Regression
Brief discussion of other regresion techniques -
 4:05
4:05Solving a System of Nonlinear Equations by Elimination
Solving a System of Nonlinear Equations by EliminationSolving a System of Nonlinear Equations by Elimination
Watch more videos on http://www.brightstorm.com/math SUBSCRIBE FOR All OUR VIDEOS! https://www.youtube.com/subscription_center?add_user=brightstorm2 VISIT BRIGHTSTORM.com FOR TONS OF VIDEO TUTORIALS AND OTHER FEATURES! http://www.brightstorm.com/ LET'S CONNECT! Facebook ► https://www.facebook.com/brightstorm Pinterest ► https://www.pinterest.com/brightstorm/ Google+ ► https://plus.google.com/+brightstorm/ Twitter ► https://twitter.com/brightstorm_ Brightstorm website ► https://www.brightstorm.com/ -
 19:50
19:50system of nonlinear equations - Newton's method
system of nonlinear equations - Newton's method
- Algebraic equation
- Algebraic geometry
- Anatol Rapoport
- Anthony Wilden
- Antilinear map
- Aperiodic
- Ball and beam system
- Bellman equation
- Bifurcation theory
- Biological system
- Black–Scholes
- Boundary values
- Béla H. Bánáthy
- C. West Churchman
- Category Systems
- Chaos theory
- Charles A S Hall
- Claude Shannon
- Closed form
- Colebrook equation
- Complex number
- Complex system
- Complex systems
- Conceptual system
- Conserved quantities
- Continuous function
- Control theory
- Cybernetics
- Derivative
- Diederich Hinrichsen
- Dimensionless
- Donella Meadows
- Dynamical system
- Economic system
- Ecosystem
- Eduardo D. Sontag
- Edward Norton Lorenz
- Elliptic integral
- Engineer
- Erwin Kreyszig
- Exponential decay
- Fluid dynamics
- Formal system
- Francisco Varela
- FuncDesigner
- General relativity
- George Dantzig
- George Klir
- Gravity
- Gregory Bateson
- Hamiltonian system
- Heinz von Foerster
- Hofstadter sequence
- Homogeneous function
- Howard T. Odum
- Human anatomy
- Humberto Maturana
- Hyperbolic sinusoid
- Ilya Prigogine
- Independent variable
- Indeterminism
- Information systems
- Integrating factor
- Interaction
- Ishimori equation
- James Grier Miller
- Jay Wright Forrester
- Kenneth E. Boulding
- Kevin Warwick
- Lagrangian mechanics
- Linear combination
- Linear map
- Linear system
- Linearly independent
- Living systems
- Liénard equation
- Logistic map
- Lyapunov function
- Map (mathematics)
- Margaret Mead
- Mathematician
- Mathematics
- Metric system
- Mihajlo D. Mesarovic
- Mode coupling
- Multi-agent system
- Murray Bowen
- Nervous system
- Niklas Luhmann
- Nonlinear optics
- Nonlinear system
- Nonlinearity
- Norbert Wiener
- OpenOpt
- Operating system
- Perturbation theory
- Phase portrait
- Physical system
- Physicist
- Political system
- Polynomial
- Polynomial equation
- Qian Xuesen
- Rational number
- Real number
- Recurrence relation
- Richard E. Bellman
- Richards equation
- Robot unicycle
- Russell L. Ackoff
- Sensory system
- Sequence
- Similar matrix
- Sine-Gordon equation
- Social system
- Solar System
- System
- System dynamics
- Systems art
- Systems biology
- Systems ecology
- Systems engineering
- Systems neuroscience
- Systems psychology
- Systems science
- Systems theory
- Talcott Parsons
- Taylor expansion
- Van der Pol equation
- Vector soliton
- Vlasov equation
- Volterra series
- Wikipedia INCITE
- William Ross Ashby
- World-systems theory
- Anatol Rapoport
- Anthony Wilden
- Charles A S Hall
- Claude Shannon
- Diederich Hinrichsen
- Donella Meadows
- Edward Norton Lorenz
- Erwin Kreyszig
- Francisco Varela
- George Dantzig
- George Klir
- Gregory Bateson
- Heinz von Foerster
- Humberto Maturana
- Ilya Prigogine
- James Grier Miller
- Jay Wright Forrester
- Kevin Warwick
- Margaret Mead
- Murray Bowen
- Niklas Luhmann
- Norbert Wiener
- Qian Xuesen
- Talcott Parsons
- William Ross Ashby
-

How to Distinguish Between Linear & Nonlinear : Math Teacher Tips
Subscribe Now: http://www.youtube.com/subscription_center?add_user=ehoweducation Watch More: http://www.youtube.com/ehoweducation Distinguishing between the terms linear and non-linear is pretty straightforward if you just keep a few important things in mind. Distinguish between linear and non-linear with help from a mathematics educator with over a decade of experience in this free video clip. Expert: Jimmy Chang Filmmaker: Christopher Rokosz Series Description: Teaching math always requires that you take the comfort level and learning abilities of your students in mind when planning lessons. Get tips specifically geared towards teaching mathematics with help from a mathematics educator with over a decade of experience in this free video series. -

Intro to Control - 4.3 Linear Versus Nonlinear Systems
Defining a linear system. Talking about the difference between linear and nonlinear systems. -

-

ODE | Linear versus nonlinear
Examples and explanations for a course in ordinary differential equations. ODE playlist: http://www.youtube.com/playlist?list=PLwIFHT1FWIUJYuP5y6YEM4WWrY4kEmIuS In this video we explain what a linear differential equation looks like, and give some examples of nonlinear differential equations. This is done by analogy with linear and nonlinear algebraic equations. We give the general form for a first order and a second order linear differential equation. -

Nonlinear Model Fitting using Excel
Using Excel's Solver tool to estimate non-linear model fitting parameters. -

Nonlinear Regression in Microsoft Excel
A three parameter (a,b,c) model y = a + b/x + c ln(x) is fit to a set of data with the Excel solver add-in. This tutorial walks through the process of installing the solver, setting up the objective (normalized sum of squared errors), and adjusting the parameter values to minimize the SSE. -

Nonlinear Systems: 3 Nonlinearity Overview
Having now laid down our foundations this is where our discussion on nonlinearity really starts. We will talk about why and how linear systems theory breaks down as soon as we have some set of relations within a system that are non-additive, which appears to be often the case in the real world, we also look at how feedback loops over time work to defy the homogeneity principle with the net result being nonlinear behavior. For full courses, transcriptions & downloads please see: http://complexitylearning.io Twitter: https://twitter.com/complexitylearn Facebook: https://goo.gl/ggxGMT G+: https://goo.gl/G6zTMg Transcription excerpt: Although it is often said that nonlinear systems describe the vast majority of phenomena in our world, they have unfortunately been designated as alternatives, ... -

Linear and nonlinear functions (example 1) | 8th grade | Khan Academy
Practice this lesson yourself on KhanAcademy.org right now: https://www.khanacademy.org/math/cc-eighth-grade-math/cc-8th-linear-equations-functions/linear-nonlinear-functions-tut/e/linear-non-linear-functions?utm_source=YT&utm;_medium=Desc&utm;_campaign=8thgrade Watch the next lesson: https://www.khanacademy.org/math/cc-eighth-grade-math/cc-8th-linear-equations-functions/linear-nonlinear-functions-tut/v/linear-and-nonlinear-functions-example-1?utm_source=YT&utm;_medium=Desc&utm;_campaign=8thgrade Missed the previous lesson? https://www.khanacademy.org/math/cc-eighth-grade-math/cc-8th-linear-equations-functions/linear-nonlinear-functions-tut/v/recognizing-linear-functions?utm_source=YT&utm;_medium=Desc&utm;_campaign=8thgrade 8th grade on Khan Academy: 8th grade is all about tackling the meat ... -

Little Tybee's Josh Martin presents: Try This! Nonlinear Pulloffs
Here's Little Tybee's Josh Martin with a new lesson video for some non-linear pulloff techniques that he likes to employ in his playing. Little Tybee is currently running a Kickstarter campaign for their new record! Check out www.littletybee.com to find out more! -

Nonlinear - Stacce
Official video. Song "Stacce", extracted from the album "Sei Mejo Te", out in 2010 on Subsound Records. Video produced by Tekla, directed by Gianluca Orrù. Assistant: Veronica Pianetti HDSL Operator: Fabrizio Cecioni www.teklastudio.com ----------------------------------------------------------------------------- "..così può capitare che 'Stacce' inizi rap, prosegua con un bridge più emo dei 30 Seconds to Mars e finisca con una cavalcata hardcore". Rockerilla -

Solve Nonlinear Equations with MATLAB
MATLAB solves nonlinear equations either symbolically or numerically with solvers such as "fsolve". This example demonstrates how to obtain a solution to sets of nonlinear equations. -

Statistics - Multiple & Nonlinear Regression
Brief discussion of other regresion techniques -

Solving a System of Nonlinear Equations by Elimination
Watch more videos on http://www.brightstorm.com/math SUBSCRIBE FOR All OUR VIDEOS! https://www.youtube.com/subscription_center?add_user=brightstorm2 VISIT BRIGHTSTORM.com FOR TONS OF VIDEO TUTORIALS AND OTHER FEATURES! http://www.brightstorm.com/ LET'S CONNECT! Facebook ► https://www.facebook.com/brightstorm Pinterest ► https://www.pinterest.com/brightstorm/ Google+ ► https://plus.google.com/+brightstorm/ Twitter ► https://twitter.com/brightstorm_ Brightstorm website ► https://www.brightstorm.com/ -

-

Nonlinear least squares example
In this screencast, we will look at an example of the mechanics behind non-linear least squares. The example focuses on fitting the Dorsal gradient in fly embryos to a bell-shaped curve. -

-

Nonlinear warfare - A new system of political control 2014 Adam Curtis
**New Adam Curtis Film** ripped from Charlie Brooker's 2014 wipe documents Putin’s adviser Vladislav Surkov Nonlinear warfare - A new system of political control. -

SAP2000 - 29 Fast Nonlinear Analysis: Watch & Learn
Learn about the SAP2000 structural analysis and design program. Copyright 2014 Computers and Structures, Inc. Visit http://www.csiamerica.com for more information. -

Linear and NonLinear Equations
This brief video describes the difference between a linear and nonlinear equation and how to apply tests to distinguish the two. Any views, opinions, data, documentation and other information presented on this video are solely those of the author and do not represent those of my employer. -

-

ANSYS Non-Linear Stress-Strain Cast Iron Tutorial - Static Structural
Advanced ANSYS Workbench Tutorial on how to apply a non-linear material model in static structural with custom stress-strain curve. This uses a command object in Workbench and Cast Iron Table data to simulate the behavior. Plastic Poisson ratio of 0.04 used and modified Mohr–Coulomb stress theory of failure for calculating the actual deformation and factor of safety. -

final solvng a single nonlinear equation in excel
Excel, solver, data table, nonlinear equation -

Solving a non-linear system of equations by graphing
http://www.moomoomath.com/solving-a-nonlinear-system-of-equations.html Solving systems of non-linear equations by graphing A non-linear equations involves graphing a parabola and a line. The point in which the parabola and line intersect is solution to your system. Always write it as an ordered pair. Video Guide 0:41 Graph y=x^2-2 y=5x-8 First graph the parabola,then the line and see where they intercept on the graph. 3:02 The solution is (2,2) (3,7) You may also enjoy... Graphing nonlinear inequalities https://www.youtube.com/watch?v=ilIeKAAKhJI Solving a nonlinear equation by graphing https://www.youtube.com/watch?v=WNkPKv0OTGI
How to Distinguish Between Linear & Nonlinear : Math Teacher Tips
- Order: Reorder
- Duration: 1:57
- Updated: 24 Feb 2013
- views: 17566
- published: 24 Feb 2013
- views: 17566
Intro to Control - 4.3 Linear Versus Nonlinear Systems
- Order: Reorder
- Duration: 5:49
- Updated: 20 Sep 2014
- views: 11939
- published: 20 Sep 2014
- views: 11939
Linear or Non-Linear Functions
- Order: Reorder
- Duration: 7:05
- Updated: 29 Jan 2013
- views: 20151
ODE | Linear versus nonlinear
- Order: Reorder
- Duration: 3:26
- Updated: 09 Sep 2012
- views: 62509
- published: 09 Sep 2012
- views: 62509
Nonlinear Model Fitting using Excel
- Order: Reorder
- Duration: 15:05
- Updated: 31 Jan 2014
- views: 55489
- published: 31 Jan 2014
- views: 55489
Nonlinear Regression in Microsoft Excel
- Order: Reorder
- Duration: 9:14
- Updated: 22 Aug 2015
- views: 3123
- published: 22 Aug 2015
- views: 3123
Nonlinear Systems: 3 Nonlinearity Overview
- Order: Reorder
- Duration: 6:47
- Updated: 05 Apr 2015
- views: 1558
- published: 05 Apr 2015
- views: 1558
Linear and nonlinear functions (example 1) | 8th grade | Khan Academy
- Order: Reorder
- Duration: 2:26
- Updated: 04 Jun 2013
- views: 66102
- published: 04 Jun 2013
- views: 66102
Little Tybee's Josh Martin presents: Try This! Nonlinear Pulloffs
- Order: Reorder
- Duration: 4:39
- Updated: 16 Mar 2016
- views: 756
- published: 16 Mar 2016
- views: 756
Nonlinear - Stacce
- Order: Reorder
- Duration: 4:15
- Updated: 26 Oct 2010
- views: 9749
- published: 26 Oct 2010
- views: 9749
Solve Nonlinear Equations with MATLAB
- Order: Reorder
- Duration: 7:09
- Updated: 03 Sep 2015
- views: 2415
- published: 03 Sep 2015
- views: 2415
Statistics - Multiple & Nonlinear Regression
- Order: Reorder
- Duration: 14:52
- Updated: 16 Feb 2014
- views: 8464
- published: 16 Feb 2014
- views: 8464
Solving a System of Nonlinear Equations by Elimination
- Order: Reorder
- Duration: 4:05
- Updated: 17 May 2010
- views: 25494
- published: 17 May 2010
- views: 25494
system of nonlinear equations - Newton's method
- Order: Reorder
- Duration: 19:50
- Updated: 02 Mar 2014
- views: 36566
Nonlinear least squares example
- Order: Reorder
- Duration: 8:50
- Updated: 12 Mar 2015
- views: 4755
- published: 12 Mar 2015
- views: 4755
Solving Nonlinear Systems with Substitution
- Order: Reorder
- Duration: 3:46
- Updated: 03 Mar 2014
- views: 2314
Nonlinear warfare - A new system of political control 2014 Adam Curtis
- Order: Reorder
- Duration: 5:08
- Updated: 31 Dec 2014
- views: 24046
- published: 31 Dec 2014
- views: 24046
SAP2000 - 29 Fast Nonlinear Analysis: Watch & Learn
- Order: Reorder
- Duration: 17:58
- Updated: 12 May 2014
- views: 25953
- published: 12 May 2014
- views: 25953
Linear and NonLinear Equations
- Order: Reorder
- Duration: 7:20
- Updated: 02 Jan 2012
- views: 33895
- published: 02 Jan 2012
- views: 33895
Solving Nonlinear Systems with Elimination
- Order: Reorder
- Duration: 7:24
- Updated: 03 Mar 2014
- views: 958
ANSYS Non-Linear Stress-Strain Cast Iron Tutorial - Static Structural
- Order: Reorder
- Duration: 15:07
- Updated: 21 Feb 2015
- views: 11832
- published: 21 Feb 2015
- views: 11832
final solvng a single nonlinear equation in excel
- Order: Reorder
- Duration: 3:26
- Updated: 30 Mar 2014
- views: 2261
Solving a non-linear system of equations by graphing
- Order: Reorder
- Duration: 3:14
- Updated: 03 Nov 2014
- views: 1770
- published: 03 Nov 2014
- views: 1770
- Playlist
- Chat
- Playlist
- Chat

How to Distinguish Between Linear & Nonlinear : Math Teacher Tips
- Report rights infringement
- published: 24 Feb 2013
- views: 17566

Intro to Control - 4.3 Linear Versus Nonlinear Systems
- Report rights infringement
- published: 20 Sep 2014
- views: 11939

Linear or Non-Linear Functions
- Report rights infringement
- published: 29 Jan 2013
- views: 20151

ODE | Linear versus nonlinear
- Report rights infringement
- published: 09 Sep 2012
- views: 62509

Nonlinear Model Fitting using Excel
- Report rights infringement
- published: 31 Jan 2014
- views: 55489

Nonlinear Regression in Microsoft Excel
- Report rights infringement
- published: 22 Aug 2015
- views: 3123

Nonlinear Systems: 3 Nonlinearity Overview
- Report rights infringement
- published: 05 Apr 2015
- views: 1558

Linear and nonlinear functions (example 1) | 8th grade | Khan Academy
- Report rights infringement
- published: 04 Jun 2013
- views: 66102

Little Tybee's Josh Martin presents: Try This! Nonlinear Pulloffs
- Report rights infringement
- published: 16 Mar 2016
- views: 756

Nonlinear - Stacce
- Report rights infringement
- published: 26 Oct 2010
- views: 9749

Solve Nonlinear Equations with MATLAB
- Report rights infringement
- published: 03 Sep 2015
- views: 2415

Statistics - Multiple & Nonlinear Regression
- Report rights infringement
- published: 16 Feb 2014
- views: 8464

Solving a System of Nonlinear Equations by Elimination
- Report rights infringement
- published: 17 May 2010
- views: 25494

system of nonlinear equations - Newton's method
- Report rights infringement
- published: 02 Mar 2014
- views: 36566
-
Lyrics list:text lyricsplay full screenplay karaoke
Kim Kardashian, Emily Ratajkowski post topless bathroom pic
Edit New York Daily News 30 Mar 2016‘Women Should Be Punished’ For Having Abortions
Edit Inquisitr 30 Mar 2016Archaeologists Find Rare Etruscan Stone From 2,500 years Ago in Florence
Edit WorldNews.com 30 Mar 2016Nobel Laureate to speak at McNair Symposium (Baylor College of Medicine)
Edit Public Technologies 30 Mar 2016UCCS Galleries of Contemporary Art hosts New Mexico-based artist Nina Elder (University of Colorado at Colorado Springs)
Edit Public Technologies 30 Mar 2016NASA Awards Contract for Atmospheric Science Research (NASA - The National Aeronautics and Space Administration)
Edit Public Technologies 29 Mar 2016ConnectOtt adds more industries for third annual event
Edit Ottowa Business Journal 28 Mar 2016A new scheme for manipulating the color of single photons — Lossless wavelength conversion of single photons in an optical fiber — (NTT - Nippon Telegraph & Telephone Corporation)
Edit Public Technologies 28 Mar 2016Soileau Named to Florida Inventors Hall of Fame (University of Central Florida)
Edit Public Technologies 24 Mar 2016DoD Announces National Security Science and Engineering Faculty Fellows (US Department of Defense)
Edit Public Technologies 24 Mar 2016False Solutions? 3 Ways to Evaluate Grand Climate Proposals
Edit Huffington Post 24 Mar 2016HUFFPOST HILL - GOP Convention To Be Brokered With HotOrNot.com
Edit Huffington Post 24 Mar 2016Prof. Chong: The Math Behind iPhone Energy Harvesters, Wall Sound Filters and Origami (Bowdoin College)
Edit Public Technologies 23 Mar 2016Vice's New Cable Network Is More Popular on YouTube Than on TV
Edit Bloomberg 23 Mar 2016Slowing Down Light with a Twist (OSA - Optical Society)
Edit Public Technologies 23 Mar 2016- 1
- 2
- 3
- 4
- 5
- Next page »







